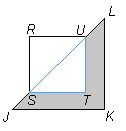
|
In the figure above, RSTU is a square
with an area of 8 and JKL is an isosceles triangle.
If JS = UL = 2, then what is the
perimeter of the shaded figure?
|
 |
Consider your answers to the following
questions, and then click Continue. |
- What is the question asking us to find?
- What information has it provided to
us?
- What shared feature will get us to
the correct answer?
|
 |
Use the information provided in
the question stem to determine the following measurements. Enter your
responses in the boxes below, and then click Continue. |
|
What is the length of side ST ?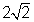
What is the length of side TU ?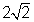
|
|
What is the length of side JK ?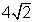
What is the length of side KL ?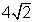
|
|
What is the length of segment JS
? 2
What is the length of segment UL?
2
|
 |
Now that we have determined the lengths
of all parts of the shaded region, calculate the total perimeter.
Select an answer and then click Continue. |
The perimeter of the shaded region consists of
the following line segments: JS + ST + TU + UL
+ JK + LK.
JS and UL equal 2: 2 + ST
+ TU + 2 + JK + LK.
ST and TU are sides of the square
and therefore equal 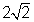: 2 +
+ + 2 + 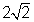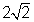JK + KL
LK and JK are legs of the larger
triangle and therefore equal :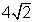
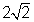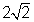2 + +
+ 2 + +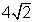
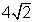
Our total perimeter is: 2 +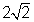
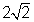+ + 2 +
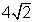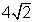+ = 4 +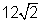 
Note that we were able to use the area of the
square provided to us in the question stem to determine the sides of the
smaller triangle. In turn, we were able to use the sides of the smaller
triangle to determine the sides of the larger triangle. This 'transfer'
of information from one piece of the diagram to the remaining figures
gave us the measurements we needed to calculate the perimeter of the shaded
region.
div>
Back Next v>
Next to display next topic in the chapter.
Video Lessons and 10 Fully Explained Grand Tests
Large number of solved practice MCQ with explanations. Video Lessons and 10 Fully explained Grand/Full Tests.