Simplify what is under the square root by factoring
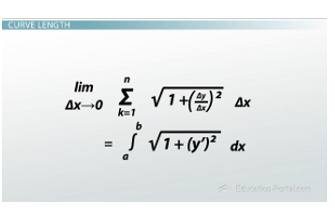
If I want to know the total distance that I walked in my trek across Disneyland, I'm going to find this distance, this square root of (delta x^2 + delta y^2), for each of these little regions, and I'm going to sum them all up. This (delta x^2 + delta y^2), all inside of a square root, looks a little bit ugly. Before I go any further, I'm going to simplify it. I'm going to factor out a delta x^2 from these two terms. I get (delta x^2)(1 + ((delta y)/(delta x))^2). Because I've got this delta x^2 as a product inside of my square root, I can pull it out of the product. My entire path becomes the sum over all of these little regions that I walked of delta x times the square root of (1 + ((delta y)/(delta x))^2). This would be my distance walked.
Using the Pythagorean theorem over a large distance isn't as accurate as using it over a much smaller distance. In fact, if I take these segment lengths to be zero, that is take the limit as delta x goes to zero of these segments, I end up getting the exact length of my path. I also end up getting the limit as delta x goes to zero the sum over all of my segments of the square root of ((1 + ((delta y)/(delta x))^2)) (delta x). When I take that limit, it's nothing more than a Riemann sum that I've taken the limit of. This is an integral. This equals the integral from the start of my path, a, to the end of my path, b, of the square root of (1 + (y`)^2)dx. This y` is just dy/dx, so that's what happens to this delta y/delta x term as delta x goes to zero. This stops being the slope of the line and starts becoming the slope of the tangent of the line.
Example Problem
Example of an integral
Let's actually find my curve length walking across Disneyland. My curve length is equal to the integral from x equals a to b of the square root of (1 + (y`)^2)dx. What is y`? My line is y = (2/3)x^(3/2). If I take the derivative of that, I get y` = x^(1/2). This is because when I take the derivative of (2/3)x^(3/2), I use the power rule, and I bring down this 3/2, so I get ((2/3)(3/2)x)^((3/2) - 1). 3/2 - 1 is 1/2. So, I end up with y`= x^(1/2). This is also known as the square root of x. I can plug that into my curve length equation, and I can also plug in my starting and ending points for x. I know that I started when x was 0, and I ended at x=4. I'm going to find my curve length as the integral from x=0 to x=4 of the square root of (1 + (x^(1/2))^2)dx; (x^(1/2))^2 is (the square root of x)^2. So, that just gives me x. My integral becomes the integral from x=0 to x=4 of the square root of (1 + x)dx.
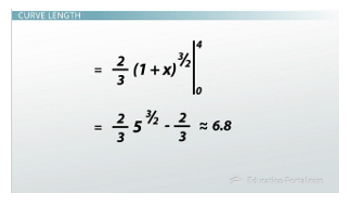
I can solve this by using a u substitution. Let's say u = 1 + x; du then equals dx. If I plug in these substitutions, my integral becomes the square root of udu, which is just (2/3)u^(3/2). Plugging in 1 + x for u and evaluating it, I get (2/3)(1 + x)^(3/2) evaluated from x=0 to x=4. If I plug in x=4, I get (2/3)(5)^(3/2). From that, I subtract my answer evaluated at x=0, which is just -2/3. This gives me about 6.8. My trek across Disneyland was 6.8, using the units that I decided.
The solution to the Disneyland trek problem
Lesson Summary
Let's review. The curve length of y=f(x) is equal to the integral from x=a to x=b of the square root of (1 + (y`)^2)dx. More importantly, though, this brings up what calculus is all about. To find that curve length, we added up a whole bunch of smaller parts, and we took the limit as that part size went to zero. This is the same thing as taking a Riemann sum and turning it into an integral, which, at the heart of the matter, is all calculus is.