Angles and Triangles: Practice Problems

Angles and Triangles: Practice Problems

Want more practice solving with angle pairs? How about more review for solving angles in triangles? Look no further. Get more practice here, and test your ability with a quiz.

Solving Angles

In another lesson, we learned about the different types of angles: consecutive interior, alternate interior, alternate exterior and corresponding. We discovered that when two lines are parallel, all of the angle pairs are congruent, except for consecutive interior angles, which are supplementary. We also learned about vertical angles, which are always congruent. Let's do some practice with these angles.

In the figure below, let Angle 5 = 30y + 31, and let Angle 9 = 22y + 55. What is the value of y?

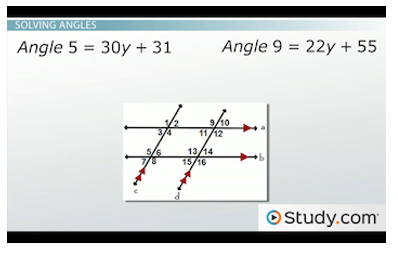
Example figure for solving angle problems

Angle 5 and Angle 9 don't match any of the angle pairs, so let's find the connection between their measures. We notice that Angle 5 corresponds to Angle 1, and Angle 1 corresponds to Angle 9. Knowing that all corresponding angles are congruent, Angle 5 = Angle 1, and Angle 1 = Angle 9. So, by the transitive property of equality, we can conclude that Angle 5 = Angle 9. By substituting the equations, we have 30y + 31 = 22y + 55. From here, we can subtract 31 from both sides to get 30y = 22y + 24, and then subtract 22y from each side to get 8y = 24. To finish, we will divide both sides by 8 to determine that y = 3.

Let's do another using the same figure. This time, let Angle 4 = 14x - 23, and let Angle 14 = 4x + 5. Find the measure of Angle 15.

Once again, these angles are not a special angle pair; so, let's find the connection. Angle 4 corresponds to Angle 12, and Angle 12 is consecutive interior to Angle 14. Therefore, Angle 4 = Angle 12, and Angle 12 + Angle 14 = 180. With this knowledge, we can replace Angle 12 with Angle 4 to get Angle 4 + Angle 14 = 180. With the equations, we have 14x - 23 + 4x + 5 = 180. Combining like terms gives us 18x - 18 = 180, and then, by adding 18 to both sides, we get 18x = 198. Last, we will divide both sides by 18 to conclude that x = 11.

Now we can find the value of Angle 15, which is vertical to and congruent with Angle 14. Substituting 11 into the equation, we see that Angle 14 = 4(11) + 5, which equals 49. Therefore, we can also conclude that Angle 15 = 49 degrees.

Solving Triangles

When working with triangles, remember that the sum of all three angles in every triangle is 180 degrees. Let's get started. In this first triangle below, let's solve for x.

Example triangle 1

For each angle, we either have a measure or an equation. For that reason, let's add all of the angles together to equal 180 degrees. Doing so, we have 40 + 10x + 20 + 20 = 180, and by combining like terms, we have 10x + 80 = 180. Next, let's subtract 80 from both sides to get 10x = 100, and then let's divide each side by 10 to finish with x = 10.

Here's another. This is triangle JKL. What is the measure of Angle L?

Example triangle 2

By having information for all three angles, we will add them together to equal 180. Remember that the square in the angle tells us that the angle measures 90 degrees. So, we can begin with 10y + 5 + 90 + 15y + 35 = 180. Combining like terms gives us 25y + 130 = 180. From here, we will subtract 130 from both sides, leaving 25y = 50. Then, let's divide both sides by 25 to see that y = 2. Now, by substitution, we see that Angle L = 15(2) + 35, which equals 65 degrees.

Let's do one more. In triangle DEF below, Angle D is two times a number, Angle E is forty more than five times the number, and Angle F is five more than two times the number. What is the measure of Angle E?

Example triangle 3

Since we don't have the exact equation for each angle, we have to use the descriptions to create them. All of the descriptions reference some unknown number. Not knowing what this number is, we will call it x in each equation. Therefore, Angle D = 2x, Angle E = 5x + 40, and Angle F = 2x + 5.

To solve, we begin with 2x + 5x + 40 + 2x + 5 = 180. Combining like terms gives us 9x + 45 = 180, and then subtracting 45 from both sides leaves us with 9x = 135. From here, we will divide both sides by 9 to get x= 15. To complete the problem, we will substitute 15 into the equation for Angle E to see that Angle E = 5(15) + 40, which equals 115 degrees.

Lesson Summary

In review, when solving with angles and lines, always begin by determining the special angle pair or the connection between the angles you were given. This will send you in the right direction for determining whether you should set the angles equal to each other or add them together to equal 180 degrees. But remember, to solve these problems in this manner, the two lines must be parallel.

When it comes to triangles, remember that all of the angles in every triangle must add together to equal 180 degrees. So, when you have information for each angle in a triangle, this is the best way to start and solve the problem.