Solving Absolute Value Practice Problems

Solving Absolute Value Practice Problems

There are many easy mistakes to make when solving absolute value equations. Learn how to avoid those mistakes here by working on examples of absolute value equations with operations on the inside and the outside of the absolute value.

Review of Absolute Values

Substituting in 3 for x in the beginning would lead to the answer 7

When it comes to solving absolute value equations, the one most important thing to remember is that they always give you two answers. We get those two answers by splitting the equation up, but how and when to split it up is where it starts to get a little more complicated and also where the mistakes start happening. In order to avoid those mistakes, it really helps to have a conceptual understanding of what 'solving an equation' means.

So let's first ask ourselves a question that you probably don't hear every day; what does a mathematical equation have in common with an onion? Now, I suppose your answer could be 'they both make you cry,' but if that was your answer, I hope this video can change your mind. Well, just like an onion has a very center and then a bunch of layers on the outside, an equation has a very center (the number) and then a bunch of operations being done to that number.

Using Onions to Solve Equations

So for example, an equation might tell us that we start with some number (lets call it x), we multiply it by 5, then we subtract 1, then we divide all of that by 2, and after we've done that, we apparently end up with 7. This can be expressed as a normal mathematical equation, or using this onion analogy where the very middle of the onion is the number we start with and each thing we do to that number is a new layer on the outside. So when we're asked to 'solve' this equation, it's simply our job to figure out what was that number in the very beginning that made it that after we do all these things we end up with 7. It's our job to peel off the layers of the onion one at a time to work our way all the way back to the very center - the x.

Solving the two resulting equations in example #2

Well the first layer I peel off is this divided by 2. After I divide it by 2, apparently I ended up with 7, which means that before I divided by 2, I must have had 14 because 14 divided by 2 is 7. So the way I can undo division is by multiplication because those are inverse operations. So by putting the little times 2 by this divide by 2, they undo each other - they cancel each other out - and over on the other side of the equation, I get 7 times 2 and I get 14. So that last step has been undone and now I have an equation with one less step.

The next layer I need to undo is the minus 1. We can undo subtraction with addition and by adding 1 to both sides of my equation, I can end up with 5 times xequals 15. Now the last layer I need to undo is multiplication by 5; I can undo multiplication with division. Those things undo and I'm left with 15 divided by 5, which is 3. This means that if we would have substituted 3 back into the very beginning and done all those different things to it, we would have ended up with 7.

Onions and Absolute Values

Absolute value equations are done the exact same way. Le'ts take a look at the example |2x+1| = 9. If we draw our onion analogy, we start with a number (x), we multiply that by 2, then we add 1, and after we've done those things, we take the absolute value and we end up with 9.

Because we're being asked to solve the equation, we have to figure out what was that number x that we plugged in way back in the beginning, and since the absolute value was the last thing to be done, it's the first thing we have to undo. I undo an absolute value by splitting it up into two separate equations; one that equals 9 and one that equals -9. Now it's just a matter of solving these two separate equations one step at a time; the first thing I have to undo is addition by 1. I undo addition with subtraction because those are inverse operations. I take one away from 9, one away from -9 and I end up with 8 or -10. Then I have to undo multiplication by 2; I undo multiplication with division and I end up with either x is equal to 4 or x is equal to -5.

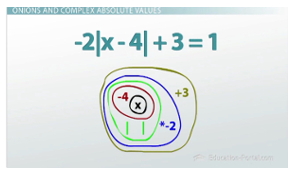
The onion for the complex absolute value problem in example #3

Onions and Complex Absolute Values

The last example will show us the final way we can complicate absolute value equations; having operations on the inside and the outside of the absolute value.

Take -2|x-4|+3=1. Looking at the onion picture for this problem shows that we start with x, we subtract 4, we take the absolute value, then we multiply by -2 and then we add 3. So the difference between this problem and the previous one is that the absolute value is not the very last thing being done to my variable, which means it's not the first thing we have to undo. A lot of people, when they see this problem, get so concentrated on the fact that there's an absolute value in it that they just think, 'split it in two equations right away!' That's not our job until we get to that layer.

First, we have to undo addition with subtraction so I minus 3 from both sides and I get -2 times the absolute value of x minus 4 is equal to -2 . Now I have to undo multiplication; I undo multiplication with division and I divide both sides by -2. Now I'm simply left with the absolute value of x minus 4 equals 1. Now the absolute value is the outermost layer of our onion, so it's time to undo it by splitting the equation into two. One where xminus 4 equals 1 and another where x minus 4 equals -1. After I've undone the absolute value, the last step to undo is the subtraction by 4; again, I undo subtraction with addition and I get my two answers that x is equal to 5 or 3.

Lesson Summary

Finding the solution for example #3

To review, an equation is just a list of steps being done to an unknown value and the answer you get after those things are done.

You can undo an absolute value by breaking the equation into two separate ones - one equal to the positive answer and one equal to the negative.

If there are no steps on the outside of the absolute value, it's the first thing you can undo by splitting it into two equations. But if there are steps on the outside of the absolute value, you must to undo those first before you split the equation up.