Graphing Absolute Value Equations

Graphing Absolute Value Equations: Dilations & Reflections

Although a basic absolute value graph isn't complicated, transformations can make them sufficiently confusing! In this lesson, you'll practice different transformations of absolute value graphs.

We'll start this lesson by recalling that absolute value graphs look like the absolute coolest guitar there is, the Flying V. Also, we can slide these graphs all around by doing the transformation that is called a translation.

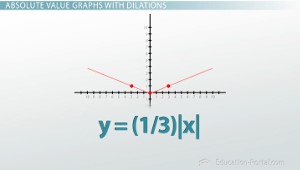
The graph of y=(1/3)|x| has a wide dilation

For example, by taking our parent graph y=|x| and changing it to y=|x-2|+3, the V gets translated two to the right and up three so that we end up with our vertex at the coordinates (2, 3). Also, remember that the left and right shift does the opposite of what you would expect. So, a -2 on the inside the absolute value actually shifts it to the right because I need to put in a +2 for x to turn the absolute value part into 0, which is where my vertex is going to be.

Absolute Value Graphs with Dilations

This is not the only kind of transformation that we often see with absolute value graphs. The second most common one is called a dilation (or a stretch or a shrink). In absolute value graphs, a dilation makes the V either wider or thinner. We accomplish this by putting a value in front of the absolute value (for example, y=2|x| or y=1/3|x|). Just like the m in y=mx+b, this value tells us the new slope of the lines in our V. Meaning that y=2|x| will start at the origin (because there is nothing being added or subtracted on the inside or the outside), but then go up two and over one each step of the way. This means it's going to be a steeper V than the normal one. If we did y=(1/3)|x|, we'd only go up one and over three each step of the way (and in both directions). That means this V is going to be wider than all the other ones we've looked at.

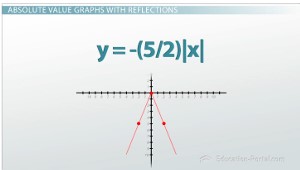
Absolute Value Graphs with Reflections

So, then the question becomes - what happens when we put a negative number in front of the absolute value (say, y=-4|x|)? Well, since the -4 is directly in front of the absolute value, which means multiplication. And, because the absolute value will always be positive no matter what we substitute in for x, (we don't multiply the positive by a negative, because it gives us a negative number) all our y values are going to be negative. So, now instead of our entire graph being above the x-axis, it will be completely below it. We call this kind of transformation a reflection because the graph of y=|x| gets reflected (like a mirror) over the x-axis to end up with y=-|x|.

Example of the reflection transformation using y=-(5/2)|x|

Quickly coming backing to the example mentioned a second ago, graphing y=-4|x| is as simple as starting at the origin (because there's no numbers being added or subtracted on the inside or the outside), going down four and then over one in each direction to find how steep or thin the graph is. Again, whether it's positive or negative, the number in front just acts as the slope - so down four over one when it's negative. We could also do y=-(5/2)|x|. We start at the origin again and go down five and over two in both directions to figure out how thin or wide our graph is.

Standard Form of Absolute Value Equations

When we combine translations, dilations and reflections into one, we end up with the standard form absolute value equation, which is y=a|x-h|+k. The a tells us the slope, h tells us how far to shift the graph left and right, and k tells us how far to shift the graph up and down.

Using this equation, we can graph things like y=-2|x-1|+3. We start by finding our vertex and going over one to the right and going up three. Then, we use the a value of -2 (the slope), which tells us to go down two and over one in both directions, and we end up with this graph. We could also do y=(3/2)|x|-5. Again, we start by finding h and k. There's nothing being added or subtracted from the x - kind of like there's a plus 0 - so we don't shift it left and right at all. We go down five to find the vertex at the coordinates (0, -5), then I use the a value as the slope. Since it's positive, we go up three and then over two in both directions to find the graph.

Lesson Summary

Graph of y=-2|x-1|+3

To review, translations slide the graph around on the coordinate plane without changing its size. Dilations make the graph wider or thinner. Reflections flip the graph like a mirror. In the standard form absolute value equation, y=a|x-h|+k, h and k give us the coordinates of the vertex, while a tells us the slope of the V. Lastly, a negative a value makes the V of the absolute value point down instead of how it normally points up.