Equation of a Line Using Point-Slope Formula

Equation of a Line Using Point-Slope Formula

It's time for a road trip to Las Vegas, and after four hours of driving at 60 mph ... Are we there yet? Learn the point-slope form of the equation of a line to help answer this age-old question.

Road Trip to Las Vegas

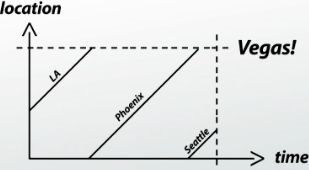
Graph of a location as a function of time

A good buddy of mine is getting married tonight in Las Vegas, so I'm going to go on a little road trip. At 2 p.m., I'm on the road to Vegas and I'm going about 60 mph. At 6 p.m., I'm still on the road to Vegas and I'm still going 60 mph. I'm out of gas, but that's another problem. Whether or not I'm in Vegas, and make it there in time for this 10 p.m. wedding, is going to depend on where I start. If I drive for 4 hours from Los Angeles, I might be in Las Vegas. If I'm trying to make it there from Miami or Seattle, I'm probably not there, especially not if I'm only going 60 mph.

Let's take a look at a graph of my location as a function of time.

I know that my velocity is my change in location divided by my change in time. On this particular graph, it's going to be the slope of any line that is connecting my location at one point in time to my location at another point in time. The slope of the line connecting these two points will be 60, but I don't exactly where these two points are. I don't know if I'm starting in Los Angeles or Phoenix or Seattle. So I don't know if I can possibly make it to this wedding by 10 p.m., because I don't know where I've started.

Point-Slope Form

Let's look at this mathematically. Let's use what's called point-slope form of an equation. This is going to relate time with my location. To use point-slope form, I need to know a point, such as my starting city, and the slope, such as my speed. For example, let's say that at 2 p.m., I'm at mile marker 40. That's my starting point. My speed will be 60 mph. I don't want to get pulled over on the way to this wedding. If I ask you intuitively where I will be after 2 hours, at 4 p.m., you might tell me that I will be at mile marker 160, if I've been going 60 mph.

The location now minus the old location equals 120 miles

How did you do that? You said that the change in time, which is the amount of time I'm driving, is 2 hours. I'm going 60 mph in those 2 hours. That means that I'm going to travel a total of 120 miles. I'm not going to be at mile marker 120, though, because I didn't start out at mile marker 0. I'm going an additional 120 miles from where I am now. So the change in location is going to be 120 miles. That's location now minus my old location. So I'm going to take 120 and add it to my current location, which is mile marker 40. In 2 hours, going 60 mph, I will go 120 miles further than I am now. I will get all the way to mile marker 160.

The Formula

We can write this very mathematically as y=y sub 1 + m(x - x sub 1). Here, our initial point, our starting location, is (x sub 1, y sub 1). That's the current time and the current location: x sub 1 is the time and y sub 1 is my location. m is my slope, delta y / delta x. That's how steep this line is, the change in y divided by the change in x. What I have here is my starting location plus my miles per hour times the amount of time, and that gives my location in the future.

Example #1

Let's do an example. Let's say that at 1 p.m., I start out at mile marker 10, and I'm going to go 72 mph. Let's use point-slope form to relate the time with my location. Point-slope form isy=y sub 1 + m(x - x sub 1). Let's start filling things in. m is the slope, so that's 72, because I'm going 72 mph. x is x; that's my time. x sub 1 and y sub 1 are my current location. So x sub 1 is the current time (this point here), which is just 1 for 1 p.m. y sub 1 is the location at 1 p.m., which is just mile marker 10. I can plug in those two numbers to get y=10 + 72(x - 1). If I want to know where I am at any given point in time, I can plug in that value for x and solve to find my location, y. So, at 4 p.m., x=4 and y is then 10 + 72(4 - 1), which is 226 miles. That's my new mile marker.

Example of point-slope formula

Example #2

All right, let's go back to this wedding problem. Let's say I'm not driving. After going 72 mph, I got too many speeding tickets, so I'm letting someone else do the driving. The wedding is at 10 p.m., and it's at mile marker 100 in Las Vegas. Currently, it's 2 p.m. and we're at mile marker 5. I leave it up to my buddy and I fall asleep in the back seat. At 6 p.m., I wake up and we're at mile marker 25. Is there any way that we're going to get to Vegas by 10 p.m.?

Here I don't know how fast we're going, so I can't use point-slope formula. But I do have two points, and I know that we're going a constant speed. I can find out what our velocity has been between 2 p.m. and now and use that to find my speed for my point-slope formula. Let's first find the slope. The two points are (2, 5), because at 2 p.m. I was at mile marker 5, and (6, 25) because at 6 p.m. I was at mile marker 25. I can find the slope of this line: delta y / delta x, which is the change in location divided by the change in time. My location has gone from 5 to 25, which is a difference of 20, and time has gone from 2 to 6, which is 4 hours. So, I've gone 20 miles in 4 hours. That gives me 5 mph. I really need to talk to this guy. We're on the freeway here. Why are we going 5 mph? Is there really bad traffic or something? That's neither here nor there. I know my slope now, and I have a point. In fact, I have two points. And I can use either one in point-slope form to find out an equation for where I will be at what time.

This can be simplified to y = 5x - 5.

So point-slope form says that y=y sub 1 + m(x - x sub 1). I'm going to use my current location as my point, so y sub 1 is 25, for mile marker 25, x sub 1 is 6, because it's 6 p.m., and m is my speed, which is a lousy 5 mph. Point-slope form gives me y=25 + 5(x - 6). I can simplify this and I end up with y=5x - 5. Where will I be at 10 p.m.? At 10 p.m., x will be 10. Plug in x=10, and I get 50 - 5, which is y=45. I will be at mile marker 45. That's nowhere close to Vegas. Vegas is at mile marker 100. When will I get to Las Vegas? This means that I need to find what value of x will give me a value of y that's 100. Let's solve 100=5x - 5. I'm going to add 5 to both sides, then divide by 5, and find that x=21 hours after I started. That means I won't get there until 9 a.m. tomorrow. Well, so much for celebrating that wedding. I probably won't even make the after party.

Lesson Summary

Let's recap. To find an equation for the line that goes through point (x sub 1, y sub 1) with some slope m, we use the point-slope formula: y=y sub 1 + m(x - x sub 1). We can use this, for example, to find out where we're going to be at any given point in time on our road trip to Las Vegas.

If, instead of having a point and a slope, we're given two points, we first can calculate the slope and then use point-slope formula. We use this in the case where, for example, I fall asleep on the road to Vegas and I only know the time and our location.