Transformations: How to Shift Graphs on a Plane

Transformations: How to Shift Graphs on a Plane

What is a transformation? Well, it's something that transforms one function into another! To see what I mean and how that looks, check out this lesson!

Transformations

Translations slide the function around

A lot of people are of the opinion that math doesn't make any sense. Some of my students even seem to think that long ago, we mathematicians met in a secret chamber somewhere and came up with all these crazy rules for no good reason other than to drive people crazy. While I can assure you that this is not the case, I do understand why people can feel this way. Teaching things like 5^0 = 1 does make it especially hard for me to defend my position that math isn't there purely for us teachers to laugh at you when you mess up.

But maybe this will help! It's a topic where we picked a word that accurately describes what it means in math. Transformations kinda, like, transform! You don't have to be an English scholar to know that this word implies one thing changing into another thing. Kinda like how a Camaro can transform into a big evil fighting robot, a mathematical function can transform into... a different mathematical function! Not as cool as the robot, I know, but at least it's simple. If it was transforming into a robot, I bet the math would be way harder.

Types of Transformations

Anyways, let's take a look at a few of the different kinds of transformations that we can have in math. First off we've got rotations. These simply take a function, maybe this one, and rotate it! We can also have reflections. These are transformations that act like a mirror and reflect a function to a new place, maybe like this, or like this! The next kind of transformation is called a dilation. These stretch or shrink the graph, maybe from something like this to this!

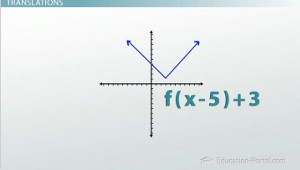
Graph of the function f(x - 5) + 3 from example #1

Translations

But the fourth and final transformation, called a translation, is the kind we'll focus on for this lesson. Translations simply slide the function around, maybe taking a function like this and moving it over here or up there! So the question becomes 'how do you accomplish this translation? How would you take this function and change it into this one?' Well, the translation I've shown you here is working in two ways. It's shifting the function up and to the right. So let's look at those shifts one at a time.

First the shift up: it looks like the new graph is three units higher than the original one. Let's see if we can make our original graph turn into this one. If we can do that, we'll be halfway and can then focus on getting it to slide over as well. Okay, so notice that this graph is really exactly the same except every point is three higher than it used to be. That means all we want to do is make every answer three more than it used to be. Instead of f(0), we want f(0) + 3. Instead of f(-2) we want f(-2) + 3. That means that, instead of f(x), we want f(x) + 3.

Cool! Now that we've gotten the function up here, let's try to slide it over to the right. But now that point needs to be five spots over to the right. So what I used to get when I plugged in 0, I now need to get when I plug in 5. So how can we plug in 5 and end up with 0? Well, what about making the function, instead of f(x), f(x - 5)? That way, when I plug in 5, I actually get f(0), which we know gives me the point I wanted. So that means that f(x) + 3 on the outside shifts it up three, but then doing f(x - 5) on the inside is actually going to shift it five to the right. That means this final graph over here must be f(x - 5) + 3.

The -3 causes a downward shift of the graph in example #2

Let's summarize what we just learned. Translations are accomplished by adding or subtracting values from the function. Adding outside the f(x) shifts the graph up, which implies that subtracting outside the f(x) would shift it down. Subtracting inside the f(x) shifts the graph to the right, which implies that adding inside the f(x) would shift it left. This is the opposite of what you might think. The way I remember it is by asking myself 'if it says f(x + 2), how would I make it zero?' The answer is, 'by putting in a -2,' thus shifting it to the left.

What this means is that if we have a function f(x - h) + k, h tells us how many units to slide the function left or right, and k tells us how many units to slide it up or down. Let's look a few quick examples.

How about this one: given that this graph is f(x), graph f(x + 1) - 3. Okay, well, the stuff inside the f(x) shifts the function left and right and does the opposite of what I'd expect it to. That means the +1 will shift everything in the graph one to the left. The -3 on the outside represents the up/down shift and follows the pattern you'd expect, which means it will pull the entire graph down three spots, which makes our new graph look like this!

One last example: The function g here is a translation of the function f. Write the equation for g in terms of f. Yikes, this one has pretty crazy directions. Let's not worry about that too much and focus on what we've learned. So, g looks basically the same as f, only it's been shifted over two to the right and up four. That means we have a -2 on the inside of the function and a +4 on the outside. Therefore, g(x) = f(x - 2) + 4

The function shifts to the right by 2 and up 4 in the final example

Lesson Summary

Let's review. Transformations of functions change one function to a slightly different one. Rotationsspin the function, reflections flip the function across a line (kind of like a mirror), dilations stretch or shrink the function and translations slide the function around.To perform a function translation, you must add or subtract values to either the inside or the outside of the f(x). Values on the inside will shift the graph in the x direction, left or right, while values on the outside will shift in the y direction, up or down. Shifting the graph up or down follows the pattern you might guess, with adding shifting it up and subtracting shifting it down, but shifting it left or right is the opposite - adding shifts it left, while subtracting shifts it right.