Completing the Square Practice Problems

Completing the Square Practice Problems

Completing the square is one of the most confusing things you'll be asked to do in an Algebra class. Once you get the general idea, it's best to get in there and actually do a few practice problems to make sure you understand the process. Do that here!

Completing the Square

Completing the square is one of the most difficult things you'll be asked to do in an Algebra class. Not only does it require you to learn a bunch of new skills but you'll also need to remember a lot of older ones as well. Before we dive right into some practice problems, let's quickly review the basics.

Completing the square turns a quadratic equation in standard form into one in vertex form. A trinomial in standard form (y = ax^2 + bx + c) can be factored into a perfect square binomial as long as the c-value on the end is equal to (b/2)^2. If your trinomial's c-value isn't naturally equal to this exact right amount, you can 'complete the square' by performing operations on both sides of the equation to force the c-value into being exactly what you want it to be.

Completing the square turns a quadratic equation in standard form into one in vertex form.

Example #1

So what does this look like in practice? Let's try changing this standard form equation (y = x^2 - 20x - 25) into vertex form by completing the square. That requires us to turn this trinomial into a perfect square binomial. For this to work, the c-value of the trinomial needs to be the exact right number: (b/2)^2. Let's check to see if this is already the right value. In this case, our b-value is -20, so -20/2 = -10 and (-10)^2 = 100, so we're not in good shape yet. Right now our c-value is -25 but we need it to be 100. Luckily this is an equation, which means we can add or subtract something from both sides of the equals sign in order to change the c-value into exactly what we want it to be.

In this case, changing -25 into 100 means that we'll have to add 125 to both sides of the equation, leaving it like this: y + 125 = x^2 - 20x + 100. At this point, as long as we haven't made a mistake, we can now factor the trinomial. Factoring requires us to look for two numbers that add up to the middle term, -20, and multiply to the constant on the end, 100. Quickly looking through the factors of 100, and then finding a pair of them that adds up to -20. So, -10 and -10 are our two winners, which means I can factor the trinomial on the right as (x - 10)(x -10). Which means the new equation will look like this: y + 125 = (x - 10)(x -10). Because the x - 10s are the same, I can combine them into one and write it as a square binomial, (x - 10)^2, leaving our equation like this: y+ 125 = (x - 10)^2. We've now completed the square! All that's left to do is move the 125 over to the other side in order for the quadratic to be in vertex form. That makes y = (x - 10)^2 - 125 the same equation as before, just expressed differently, and it's our final answer.

The final answer is the same equation as before, expressed differently.

As a note: Completing the square is actually often used to solve quadratic equations as well. If instead, this problem had not said complete the square, but solve, and instead of y we had a zero, we would have gotten an equation that looks like this: 0 = (x - 10)^2 -125. Now we can simply use inverse operations to solve for x by undoing the 125, undoing the square with a square root and undoing -1 with a +1 to get our answer, like this: 1 +/- the square root of 125 = x. This is often what you'll use completing the square for - a way to solve a quadratic equation that's not factoring or the quadratic formula.

Although that may have seemed like a pretty long process, it was actually a straightforward example. Although the other problem we'll do during this lesson will basically be the same thing, it will have a few complexities that make it a little bit trickier.

Example #2

First off, the directions are going to be a little bit different: If y = 6x^2 + 30x + 18 is expressed in the form y = a(x- h)^2 + k, what is the value of k? Different words, but same idea. Now though, once we've changed it to vertex form, the answer is simply the k-value and not the entire equation. But that's fine, same thing.

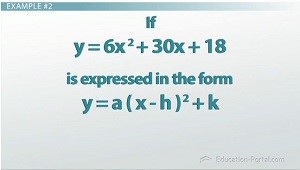
What is the value of K?

Alright, so let's begin. Right away there is something else that is different though. Our a-value isn't 1. Hmm, okay. Well, looking at the vertex form here, I see that the a-value is on the outside of the parentheses. That means we'll have to factor it out of the trinomial before we can turn it into a perfect square binomial. Thankfully, each term in this trinomial is divisible by three, so it won't be too bad, but sometimes this actually won't be the case. So, I need to divide out a 6 from each term on the right-hand side. Doing that leaves us with this: y = 6(x^2 + 5x + 3). Now we're ready to check to see if our c-value follows the pattern. It's going to need to be (b/2)^2, which in this case will be (5/2)^2. Uh oh, another hurdle. We're going to have a fraction or a decimal this time. Last time, our b-value was an even number, so dividing it by 2 wasn't a big deal. But this time it's odd, making things a little bit trickier but still doable. (5/2)^2, or 2.5^2, gives us 25/4 (if we want to use fractions) or 6.25 (if we want to use decimals). So, now we know what our c-value needs to be. We look at our problem as it is, and we realize it is not quite there yet (3 is not the same as 6.25).

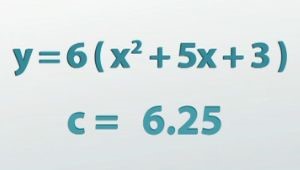
Turn the current c value into 6.25.

Turning our current c-value, 3, into 6.25 will require us to add 3.25 to it, but we're going to have to be careful. When we add 3.25 to the trinomial on the inside the parentheses, we're actually adding 6 * 3.25 to that whole side of the equation. Since whatever we do to one side of the equation we have to do to the other, we need to actually add 19.5 to the left, not just 3.25. Doing that leaves us with this: y + 19.5 = 6(x^2 + 5x + 6.25). Alright, we're making progress! Our trinomial is finally ready to be factored, and this time let's use the shortcut. As long as we've done everything right, x^2 + 5x + 6.25 should be factored as (x + 2.5)(x + 2.5) because that 2.5 is half of our b-value, 5. Then compressing the factored form as a square binomial, (x + 2.5)^2, and then undoing the 19.5 to write the equation in vertex form, like so, y = 6(x+2.5)^2 - 19.5, allows us to answer the question 'What is k?' Well, k is the constant on the end, so k is -19.5.

This last example is close to as tough as it gets for completing the square. Hopefully, you feel confident enough to try the examples in the quiz following this video all by yourself.

Lesson Summary

To review, to complete the square, we need to change the c-value in our trinomial to be (b/2)^2. If you have an a-value that is not equal to 1, you'll need to first factor that value out of each term in the trinomial before you can proceed. This also means that you'll have to be careful to multiply by this factor when deciding how much to add to both sides when making your c-value the correct amount. If you end up with an odd b-value in your trinomial, you'll end up with fractions or decimals in your answer, but that's okay!