To factor a quadratic, it's really nice not having a negative x2 term, so let's divide that part out first. Pulling a negative from each term leaves us with this equation instead. Now we can begin to look for the two numbers that have a product of -16 and a sum of -6. The reason we're doing that is all about factoring. So if you're struggling with factoring, you should probably go back and check out a previous lesson to get the basics. Finding a pair of numbers that satisfy these two equations will be easiest if we write out the different factors of 16 and look for the pair of them that can add or subtract to -6. Doing that makes it look like 2 and -8 are our winners. We can therefore rewrite our equation as this: (0 = -(x - 8)(x + 2)).
But why did we do that? How has that gotten us any closer to solving this equation for x? I still have two of them; I can't get one of them by themselves. Well, what we now have is a product that equals zero. This allows us to use what is called the zero product property, which says that anytime a product equals zero, at least one of the things we are multiplying must be zero. Another way of saying that is this: the only way to multiply two things and end up with zero is by having one of those two things be zero itself. What this allows us to do is split the equation up and say that either x - 8 or x + 2 is equal to zero. Now that the xs are separated, we can again use inverse operations to solve for them. Undoing -8 and +2 tells us that the two places where our bird will hit the ground are at x = -2 or x = 8.
Well, in our game here that -2 answer might happen if the slingshot broke, but we're more concerned with the answer of 8. And it looks now, if our bird flies out to 8, it will go right over the pig we're trying to hit, so making this shot would be a waste of a bird.
Example #2
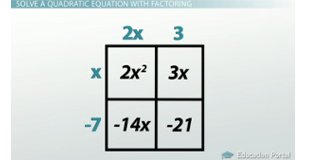
Use the area method to find the factored expression in example #2.
But if we slightly adjust it, maybe aiming up a little bit so that our bird flies higher in the air but doesn't go as far, we are told it will now fly the path of this parabola y = -2x2 + 11x + 21. Let's see if this does what we want it to.
We'll again substitute in y = 0 in order to find out when our bird will hit the ground, and then factor the quadratic to separate the xs and allow us to use the zero product property. We again begin by taking out the negative in order to help us factor, leaving us with this. We then need to find a pair of numbers whose product is -42 (the first term times the last term) and again, whose sum adds up to -11. Writing out the factors of 42 and comparing the pairs of numbers makes it appear that 3 and -14 are our winners. But since we have a 2 in front of our x2 term, we'll need to use the area method to figure out exactly how this all factors. Putting the two values we just found in one diagonal of our box as coefficients on x's, with the 2x2 and the -21 in the other diagonal, allows us to work our way to the outside and find our factored expression. We do this by finding the greatest common factor in each row and column.
In the far left column, both terms have a 2 and an x; in the right column both terms have a 3. In the top row, both terms only share an x; and in the bottom row, both terms only share a -7. This makes the factored form of (2x2 - 11x - 21) the same as 2x + 3 times x - 7. Again, for more help on factoring, check out the previous video.
Now that we've factored the quadratic, we can use the zero product property to split up the xs and say that either 2x + 3 = 0 or x - 7 = 0. A few quick inverse operations later tells us that our bird will hit the ground at either 7 or -3/2. Our -3/2 answer doesn't concern us too much, but it looks like having our bird land at 7 is exactly what we want. We can fire this bird feeling confident that we'll hit it on our first try and pass level one.
Congratulations! You've just passed level one of 'Furious Fowls!' You can now move onto level two, where things are going to get a little bit harder.
Lesson Summary
Before you do that, though, let's quickly review what you've learned from level one. If you are trying to find when an object launched into the air will hit the ground, you must set the quadratic equation equal to zero and solve it.
Solving quadratic equations with only inverse operations won't work because there are x's and x2's. What we need a way to break them up first. One way to do this is with factoring and then using the zero product property. This allows you to set up two separate equations that can be solved with inverse operations to get our two separate answers.