How to Solve and Graph an Absolute Value Inequality

How to Solve and Graph an Absolute Value Inequality

Taking two skills and combining them to make a more complicated problem is a classic tactic in mathematics. If you feel confident with absolute values and inequalities, see if you can tackle them with their powers combined here!

Reviewing Inequalities and Absolute Value

Mathematicians love to experiment, so it makes sense that once they came up with the separate ideas of absolute values and inequalities they thought 'let's see what happens when we put them together!' But before we try to figure that out for ourselves, let's quickly go over what those two things are individually.

Inequalities mean 'greater than' or 'less than' and are good for expressing situations where something can be at least or at most something else. We can have 1-variable inequalities that have graphs on number lines and 2-variable inequalities that have graphs on the coordinate plane. We can solve inequalities, just like equations, with inverse operations as long as we remember the one extra rule: you must flip the inequality symbol around when multiplying or dividing both sides by a negative number.

Example of an or compound equality

Absolute values are important when we only care about the magnitude of a number, or how 'large' it is regardless of whether it's positive or negative. Because of this, the absolute value turns all numbers positive whether or not they were positive to begin with. Graphing absolute values gives us what look like Vs, because when the line would normally keep getting negative, the absolute value instead bounces it back up to the positives.

When we solve absolute value equations, we can still use all the same inverse operations to get variables by themselves, but we must remember that the way to 'undo' an absolute value is by splitting the equation up into two new ones: one that equals the positive and one that equals the negative. Therefore, it's usually the case that absolute value equations give us two answers.

1-Variable Absolute Value Inequalities

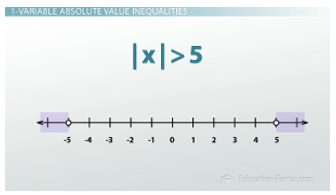
So now that the review is out of the way, let's combine those two skills into one as simply as we can. Solve and graph | x | > 5.

Without learning anything new, let's try to solve this problem just like we would before. If I'm being asked to solve, and I've got an absolute value, I need to undo it by splitting the inequality up. This leaves me with two inequalities, x > 5 or x > -5.

Well, that wasn't too bad. I am now left with a compound inequality because I have two inequalities in one, but that's okay. Let's check our answers to make sure it's really that easy.

x > 5 says that numbers like 6 or 100 should work in the original problem, so we can substitute those in, take absolute values, and, sure enough, they check out.

Example of an and compound inequality

Okay, now x > -5. That says that numbers like -4 or 1 should work. So we can substitute those into the original problem, take absolute values and... huh, they don't. Since the absolute value makes them positive, 4 and 1 don't work because their magnitude isn't as big as 5. So what we really need is actually 'large' negative numbers, like -10 or -100, because then the absolute value would change them positive again and we'd be in business. So instead of x > -5, what we actually need to have is x< -5 because numbers like -10 and -100 are technically less than -5, although they are 'larger' or higher-magnitude numbers.

What we've just discovered is the Golden Rule of solving 1-variable absolute value inequalities. When splitting the inequality into two new ones to undo the absolute value, we need to flip the sign on the one we set to the negative. I personally remember this by thinking about it like the multiplying and dividing by a negative rule, where you have to flip the sign. It's kind of similar.

Anyways, now that we've got our correct answer as x > 5 or x < -5, we can graph the compound inequality using our previous knowledge.

Doing one inequality at a time and putting an open circle at 5 because it's just 'greater than' and not 'or equal to' and drawing an arrow to the right to indicate all the numbers bigger than 5 takes care of half of the graph. Then putting another open circle at -5 and drawing our arrow to the left to indicate the smaller numbers takes care of the rest.

Use translation skills to graph the boundary for the equation

Looking at the graph shows us that what we have is an 'or' compound inequality because the two arrows are going in opposite directions. That means that numbers bigger than 5 or ones smaller than -5 will both work in our original inequalities.

Absolute value inequalities can also give us 'and' compound inequalities as well. For example: solve and graph |x| < 5

Now, instead of looking for very 'large' numbers that will need to be bigger than five after they've been turned positive, we are looking for a select group of 'small' numbers that will still be smaller than five even after they have been turned back positive.

Using our new skill of flipping the inequality symbol when undoing an absolute value gives us our solved inequality as x < 5 and x > -5. Remember I had to flip it.

The reason this is an 'and' compound inequality is that in order for our solution to work, it has to satisfy both conditions. Not only does it have to be less than 5, it also has to be greater than -5. It won't work if only one of those things is true and not the other one. Take, for example, 10: 10 is greater than -5, but it is not less than 5. Therefore, when we substitute 10 back into the original inequality, you'll find that it doesn't work. Any compound inequality where both conditions have to be true in order for your solution to work is an 'and' compound inequality. Once we graph the inequality, we get this, and we see that only the 'small' numbers between -5 and 5 will still be less than 5 after being changed positive.

2-Variable Absolute Value Inequalities

Lastly, we can also graph 2-variable absolute value inequalities, say: y < |x-2| + 1.

Just like other 2-variable inequality graphs, we need to begin by graphing the boundary line right where y = |x-2| + 1.

Using a dotted line shows it is not part of the solution

This requires us to use our translation skills: use the -2 on the inside of the absolute value to shift the vertex to the right and the positive 1 on the outside to move the vertex up 1. Because there is no variable in front of the absolute value, we can assume there's a little imaginary number 1 in there, and that tells us the slope of the V is 1, which means from my vertex, I go up 1 and then over 1 in both directions to find the V.

We now need to decide which side of the V is the 'greater than' side and which is the 'less than' side. Substituting in the origin (0,0) to the original inequality is still a good strategy, and doing so gives us 0 < |0-2| + 1. Simplifying this down gives us 0 < 3, which is a true statement. Therefore, the area of the graph that the origin is in is the part of the graph that we should shade. This means that we shade everything on the outside of the V - on the left, below it and to the right.

Lastly, because the inequality was strictly 'less than' and not 'equal to', we make the V a dotted line to indicate that it is not part of the solution. After all the steps are done, we are finished and we have this graph as our answer.

Lesson Summary

When solving 1-variable absolute value inequalities, there is one new rule to remember: when undoing an absolute value by splitting the equation into two, you must flip the inequality symbol on the inequality that is set to the negative (kinda like the whole 'flipping the sign when multiplying or dividing by a negative' thing).

1-variable absolute value inequalities give us compound inequalities.

An absolute value that is greater than something gives us an 'or' compound inequality, whereas an absolute value that is less than something gives us an 'and' compound inequality.

Graphing 2-variable absolute value inequalities has no new rules to remember, and your answers should look like Vs, with either the inside or the outside of the V shaded.