How to Calculate an Arithmetic Series

How to Calculate an Arithmetic Series

In this lesson, you'll learn how to sum up any arithmetic sequence without having to add up each and every term. You'll never guess the circumstances that led to the discovery of this marvelous formula.

What is an Arithmetic Series?

The pattern Gauss discovered for arithmetic series

An arithmetic sequence is a pattern of numbers that go up by the same amount each time, like 1, 2, 3, 4, 5, … which means that an arithmetic series is what you get when you're asked to add up all the entries within this pattern, like 1+2+3+4+5+…

Well this is exactly what Karl Friedrich Gauss's teacher asked him to do when he was just a boy in elementary school. Apparently, the teacher had had a long day and decided to give the class a little problem that would keep them busy for a while, so that he could maybe take a quick nap.

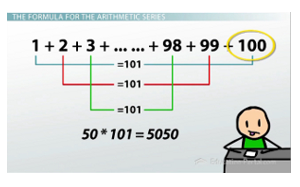
He instructed them to add up all the numbers between 1 and 100 and then sat down at his desk and put his head down. But much to his dismay, a few minutes later a young Gauss walked up to him and told him the answer, 5050. This must be a joke, thought the teacher, I don't even know the answer to this myself, how could this kid have gotten the answer so fast? It turns out that little Gauss had stumbled upon the formula for any arithmetic series.

The Formula for any Arithmetic Series

The formula for any arithmetic series

What Gauss noticed is that if he paired up the first and last terms, 1 and 100, he got 101. But if he did the same with the second and second-to-last terms, 2 and 99, he ended up with the exact same thing, 101. If he did the same with 3 and 98, that was still 101. He kept making more and more pairs and noticed that they kept giving him 101. He decided that, since there were 100 terms in the series, there'd be half as many pairs, or 50 pairs of 101. Therefore, he was able to find out that the series 1+2+3+4+….+98+99+100 was just 101x50=5050.

It turns out that this trick will work for any arithmetic series. Simply pair up the first and the last term (a_1 + a_n) and multiply by however many groups you have (n/2), and you get your sum! We therefore have the following formula written in summation notation. We start with the first term and go to n, where n is the number of terms in our series, and we're doing this for some arithmetic sequence a_n. What we get is (n/2), which is the number of terms divided by 2, times the sum of that first and last term (a_1 + a_n). So, that gives us (n/2)(a_1 + a_n), which is our formula for any arithmetic series.

Solving the Michigan Stadium Problem

The sequence for the number of seats in each row at Michigan Stadium

Now that we know that shortcut, we're finally ready to solve a problem we introduced in an earlier video: how many seats are in one section of Michigan Stadium? We said that there were 35 seats in the first row and four more in each row behind. That made the number of seats in the nth row a_n = 4n + 31because the zeroth row, if there was one, would only have 31 seats, and the common difference was 4. What we're really asking is what is the sum of a series that starts at the first row and goes through the 96th row while following the sequence 4n + 31? Again, the number of seats in this sequence is represented using summation notation just like this.

So, how do we use our formula? Well, first we have to decide how many groups there are going to be. There are a total of 96 rows, so we have to divide that by 2 since we're taking pairs, so that's the n/2 part of the formula. Now we have to find out how much each group is equal to. I find that by taking the number of seats in the first row and the number of seats in the 96th row before adding that together. For the first row, I can plug in 1 into that 4n + 31 formula, which gives me 35. For the 96th row, I plug in 96, which gives me 415. That gives me 450 for each group. 96/2 gives me 48 groups. So, 48*(450) = 21,600, and we now know that there are 21,600 seats in each section of Michigan Stadium!

The solution for the Michigan Stadium problem

Lesson Summary

Adding up arithmetic series can be made a lot faster and easier by pairing numbers from the beginning of the series with numbers from the end. Doing this gives us the formula, starting from the first term and going to the nth term, (n/2)(a_1 + a_n) where nrepresents the number of terms in the series

To try some more arithmetic sequence practice problems, you should check out the practice problem video that should be posted a few lessons after this one.