How to Graph 1- and 2-Variable Inequalities

How to Graph 1- and 2-Variable Inequalities

So an inequality is just an equation with a less than or greater than symbol. But what is the difference between 1 and 2 variable inequalities? What does have a greater than or equal to symbol change? When do you use a number line instead of a coordinate plane? Get those answers here!

Graphing a 1-Variable Inequality

All right, so you know that an inequality is an equation with a greater than (>) or less than (<) symbol instead of an equals sign (=). You know they're nice for expressing situations where there can be at least, or no more, than some amount. But what is it that you really need to know? What are you going to be asked to do, and how should you do it? That's what this video is going to focus on.

The thing is, inequalities are a broad enough topic that they can appear in lots of different places; from quadratic equations to cubic, logarithmic, exponential, rational, the list goes on and on. So, in order to limit the amount of other things you have to worry about, we're going to introduce inequalities in the easiest place you'll find them: linear equations.

Line graph for x is less than 3

So, here we go with a linear inequality:

Solve and graph 4x + 3 < 15.

It's an inequality because it has a < symbol, and it's linear because the x isn't being raised to any fancy exponent. We also know that any time we're asked to solve something, it's our job to figure out what value makes the statement true. And just like linear equations, linear inequalities can be solved using inverse operations to get the variable by itself.

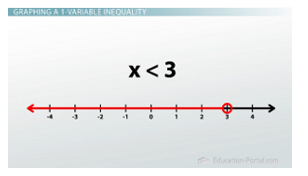
Undoing plus 3 with minus 3, and then undoing times 4 with divided by 4 leaves us with our solved inequality x < 3.

So, instead of just one value that will make the original statement true (like an equation), here, any number that is less than 3 will work. That means there are tons of right answers: 2, 1, 0, -5, -10, -1,000, negative...whatever. So instead of making one gigantic list, graphs are used to represent all the possible solutions in one clean picture.

When I think about making a graph, I have to think about what kind of inequality this is.

This is a 1-variable inequality because there's only one variable, x. So, that means our graph will only have one axis, and therefore be called a number line. We often start by putting 0 on the number line, then working our way over to the point 3, we put a circle there to indicate that is the boundary point between the good answers and the bad ones. We then draw an arrow to the left to indicate that all the good answers are smaller than 3. And we're done!

Line graph for x is greater than or equal to 6

If instead our problem would have had a less-than or equal-to sign instead, we would also want to fill in our circle at 3 in to indicate that we want to include that number -the boundary point - in our 'good list' of possible solutions.

So far, inequalities are no different to solve than equations. But there is one difference. The example solving 5 - x is less than or equal to -1 will show that difference.

Well okay, undoing the 5 with subtraction and then undoing the -1 with division leaves us with x is less than or equal to 6, great right? Uhhh, not so much. Less than 6 you say? Okay, so 0 should work right? But wait, when I put 0 in for x, I get 5 is less than or equal to -1, that's definitely not true. So, what happened?

Well, when we divided both sides by -1 to get rid of the negative in front of the x, what we were really doing was making the x and the -6 switch sides of the inequality. When this is an equation and there's and equals sign, this is no problem; x = 10 is the same as 10 = x. But if it's an inequality, doing this changes the sentence we have; x > 10 is different than 10 > x.

Therefore, there is one Golden Rule about inequalities that make them different than equations. Anytime you multiply or divide both sides of the inequality by a negative number, you must flip the inequality symbol around.

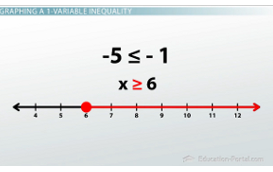
If we would have used this rule the first time, our answer would have instead said x is greater than or equal to 6. Just to make sure that works out, let's check it real fast. So, 10 is bigger than 6, so if we substitute 10 into our original equation, 5 + 10 = -5 and -5 is less than or equal to -1, so we're in good shape.

Graphing our solution now simply requires us to draw a number line, work our way over to 6, put a circle there, fill it in because it's 'or equal to', and then draw an arrow to the right, indicating anything bigger than 6 is good to go.

The boundary line is where y = 2x – 5

Graphing a 2-Variable Inequality

The last example for this video will be a 2-variable inequality. I bet you can guess what is going to be different about this one. Instead of just xs, we'll have xs and ys!

Maybe something like, graph y < 2x - 5.

Just like in the first two examples, we need to find the boundary where our bad answers end and our good answers begin. But because we now have two variables, our graph will have two axes. This means that the boundary is no longer a point like before; it's a line.

And this boundary right in between where y goes from being less than to greater than is where y = 2x - 5. I begin at the y-intercept, -5, and I use the slope to go up 2 and over 1 and I find my line right where y = 2x - 5.

So, what we have here is the boundary where y = 2x - 5, but we want to know where it is less. The best way to determine which side of the boundary is the less than side is by picking a random point, plugging it in, and seeing if it works in our inequality.

The origin, (0, 0), is often a good one to try because plugging in 0s make a lot of the math easy, so going back to our original inequality and substituting in 0s gives us 0 < - 5, which is most certainly not true. That means that (0, 0) does not live in the 'less than land,' which means it lives in the 'greater than land;' therefore, the remaining section of the graph must be what we're looking for, and y < 2x - 5 is the area underneath the boundary.

Graph for the inequality y is less than 2x – 5

But also like the 1-variable problem, we need to indicate whether or not the inequality had an 'or equal to' on it. This example did not, so we need to make it clear that the boundary line where it's equal to is not part of the answer. We'd like to just erase it, but then we wouldn't know where the 'less than land' ends and the 'greater than land' begins, so instead we make the equal line a dotted line. If the problem would have had the 'or equal to' we would have simply left the line as it was and been done.