This says that the solution set is all values of x, such that x is less than 80 or greater than or equal to 90.
Moving on, the second notation we can use is called interval notation. This notation is especially compact and used more often than set notation for expressing solution sets of compound inequalities. For example, the answer to our 'and' compound inequality could be expressed using interval notation like this: [80,90)
The first number is the lower bound, and the second is the upper bound. The opening bracket, [, indicates that 80 is included in the solution and is equivalent to saying greater than or equal to. The closing parenthesis on the other end, ), indicates that 90 is not included in the solution and is the same as saying less than.
Expressing the solution to 'or' compound inequalities is a little more complicated because there are two intervals in the solution instead of one. If we expressed the solution to our previous example of how not to get a B, it would like this: [0, 80) U [90,100]
The symbol, U, in the middle stands for union and means that both sets are part of the solution. The first set says that anything from 0 up to, but not including 80, is not a B, and the last tells us that anything from 90 to 100 is also not a B. Again, the numbers tell us the bounds, and the brackets and parentheses tell us whether the bound is, or is not, part of the solution.
System of Inequalities
Just like we can have multiple 1-variable inequalities in a single problem, we can have multiple 2-variable inequalities in a single problem as well. When this happens, it's called a system of inequalities.
We can graph a system of inequalities by working with one inequality at a time. For example, what if we were asked to find the area of the graph where both of the following inequalities were true?
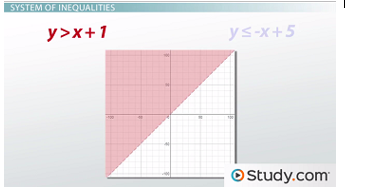
• y > x + 1
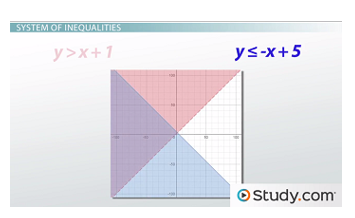
• y = -x + 5
We would begin by graphing the first inequality, y > x + 1, on our coordinate plane, getting this.
We could then graph the second inequality, y = -x + 5 directly on top of it to get this.
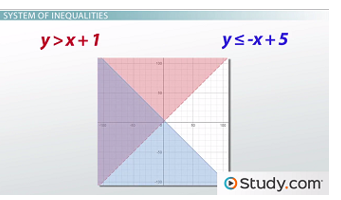
Since we want to know where both inequalities are true, we're looking for the area where the shading overlaps, leaving our answer as the purple area in this graph.
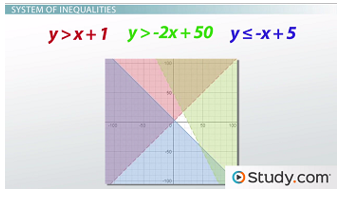
There are times when a system of inequalities will not have a solution. Let's expand our previous example by adding a third inequality: y > -2x + 50. We want to know if there is a set of solutions for which all three inequalities are true. As we can see from the graph, there are several places where the shading for any two of the three inequalities overlaps, but no single area where all three overlap. That means that there is no solution to this set of three inequalities, which is a perfectly valid answer!
Lesson Summary
To review, compound inequalities are problems with two 1-variable inequalities in them. If an answer must satisfy both inequalities, it is called an 'and' compound inequality, but if satisfying one of the inequalities is enough to be a solution, then it is called an 'or' compound inequality. There are two mathematical notations commonly used to describe the solution sets of compound inequalities: set notation and interval notation.
Systems of inequalities are problems with two or more 2-variable inequalities. You can graph a system of inequalities by working with one inequality at a time.