What Is Domain and Range in a Function

What Is Domain and Range in a Function?

The domain and range are the possible outputs and inputs of a function. In this lesson, learn about what might restrict the domain and how to figure out the domain and range from a graph.

Enjoy More Slurp

Earlier, we talked about how a function is like a soda machine. By putting in different combinations of money (the inputs), you can get out different kinds of soda (the outputs). Mathematical functions are basically the same. You can input different numbers, and you get new numbers back out. But there is one aspect of functions that hasn't been talked about yet: domain and range.

Let's think about this in terms of the soda machine. There are different kinds of ways you can pay for your soda. You can use dimes, quarters, one dollar bills and some machines will even let you use your credit or debit card now. But what happens if you try to use a penny? To be honest, I'm not actually sure. I'd guess that it either spits it back out at you or just steals it and doesn't count it towards your soda. Either way, pennies don't work in the machine. That means that the domain of this soda machine is only {dimes, quarters, one dollar bills, credit cards and debit cards}. These are the possible inputs - the only things that will work in the machine.

But along with there being a limited number of things you can put into the machine, there are also a limited number of things you might get back out. No matter how you pay, there is no way you are going to get a cheeseburger back out of that machine! Let's say that the only possible soda's you can get from this machine are regular, diet and lemon-lime. That makes these three things, the range. The range of any function is the collection of possible outputs. Now that we've got an idea of what the domain and range are, let's go ahead and take a look at some real mathematical functions.

Domain & Range from Values Alone

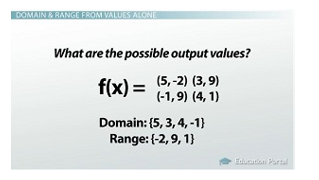
The domain and range values for the function

We'll start with an easy, simple function like this one: f(x) = (5,-2) (3,9) (-1, 9) (4,1). This function doesn't have a rule; it's simply a collection of input and output values. For example, it tells us that when you input 5, you get -2, or when you plug in 4 you get 1.

So, what is the domain of this function? Another way of asking that question is: what are the possible input values? Well there are only a couple: 5, 3, 4 and -1. That's it! What about the range? This is the same as asking what the possible outputs are. In this case, that happens to be only -2, 9 and 1. Although two different inputs both give you 9, you don't have to list it twice when writing out the range.

Graph Squishing to Find Domain & Range

We can up the difficulty a little bit by asking to determine the domain and range from a graph, maybe this one. Now, when we are thinking about the domain (or the inputs), we need to look for the x-values on this graph. Is there anywhere on the x-axis that this graph does not go? I usually answer this question by imagining the whole graph being squished down onto the x-axis, maybe like this. Now the question becomes: what parts of the axis have squished bits of the graph on it? Well assuming that this graph would continue on forever to the left and forever to the right, it looks like the whole thing has squished graph on it. This means that the domain of the function is everything, or all the real numbers.

To find the range, we'll do a pretty similar thing. Except, this time, we're concerned with the output values - the y-axis. That means we squish the graph this way, and again, look to see where the graph ended up. Now though, the entire axis is not covered. It's only covered right in this area, the middle, between -1 and +1. That means that the range is not all the real numbers, and is only the values of y contained between -1 and +1.

Graph with domain of

Finding Domain & Range from Rules Alone

The final exercise we'll look at, and probably the most difficult one we'll do, requires you to determine the domain and range from only looking at the rule of the function. These are going to be hard because there isn't a method I can teach you that will allow you to do every problem. Instead, they require you to have a good conceptual understanding of algebra and functions. But there are a few general rules I can lay out for you.

General Rules: Domain

First, in terms of the domain, there are a few main rules that you are not allowed to break. Dividing by zero is one of the no-no's. So, anytime you see a function that has an x in the denominator, there is going to be at least one value that can't be included in the domain. For example, in the function shown here: g(x) = 3 / (x + 2), x = -2 would give you a zero in the denominator. That makes the domain of this function any x except -2.

Another no-no is taking square roots of negative numbers, because those numbers don't actually exist! So again, if you see the square root of an x, you know that there will be some values excluded from the domain. As you can see here, this function h(x) = 3 times the square root of (x - 5) will have a domain of only xs bigger than 5, because any x smaller than 5 will give you a negative number inside the square root.

The final restriction on the domain we'll go over has to do with logarithms. While I could launch into a rather long and confusing explanation of why this is the case, it's probably best for you to just take my word for it when I say that we cannot evaluate logarithms of zero or any negative number. That means that the domain of this function r(x) = log(-2x) does not include any positive x-values. Only when we plug a negative number into this function, do we end up taking the log of a positive number.

Example of a function that stays positive

General Rules: Range

Now, thinking about the range, there are again a few types of functions to look out for. Maybe the easiest to see are the functions which have x raised to an even number power like: x^2, x^4 or x^6. The graphs of all of these functions look something like this, which might help you see that these functions stay positive, because even multiplying a negative number by itself an even number of times will turn it positive again. For example, (-3)^2 = +9 , but then (-3)^3 turns back negative and you get -27. But then if we go up to (-3)^4, we, again, get +81. Therefore, these even numbered exponent functions will have ranges of only positive numbers.

Be careful though. The function f(x) = -x^6 will actually have a range of all the negative numbers because the negative sign in front of the x^6 will flip the whole graph to below the x-axis, making instead of all the positive values, all the negative values our range.

It's little tricks like this that make it pretty hard to list out all the different ways the range can change just in one lesson. But other functions you need to look out for are square roots, absolute values and exponentials. All of these functions will normally have ranges that are made up of only positive values, but again, tricks can change this slightly.

Lesson Summary

The domain of a function is the collection of all the possible inputs while the range is the collection of all the possible outputs. Finding domain and range from a graph can be accomplished by imagining the graph getting squished down to either the x- or the y-axis, respectively. Whatever parts of the axis the graph has been pressed down onto are the entries for the domain or range.

Finding the domain and range from just the rule can be tricky, but there are a number of general rules to follow. Essentially, you should just be careful of any of the functions you see here, but if you don't see any of these things there's a good chance that your answer is simply, all the real numbers.