Compound Event in Math

Compound Event in Math Definition & Example

This lesson will define compound probability and how it differs from other types of probability. We'll go over different strategies for solving compound probability problems. After the lesson, you can take a brief quiz to check what you learned.

Compound Event Defined

Suppose that your friend Gary misses the bus to class half of the time. We could say that there is a 50% chance that he will miss the bus today. Gary also forgets his homework for 1 out of the 5 classes each week. We know the probability of each even occurring separately, but what if we wanted to find the probability of him missing the bus AND forgetting his homework on the same day?

When we use the term compound event in math, we are referring to the probability of two or more events happening at the same time. In our example, the two events are 1) Gary missing the bus and 2) Gary forgetting his homework. Written mathematically, the probability of this compound event would be:

P(missing the bus, forgetting homework)

Notice that each event is written in parentheses separated by a comma. The P tells us that we are calculating the probability of the events in parentheses.

So how would we find P(missing the bus, forgetting homework)? There are several strategies that can be used to solve problems with compound events. This lesson will show you how to use three common methods.

First, we will use a table to help us see all of the possible outcomes. Next, we will use a tree diagram, which maps out all of the outcomes. Both of these methods are excellent ways to understand the problem in a visual way. The third method involves a formula. Once you are comfortable with the concept of compound event probability, you may prefer to use the formula, which is usually more time efficient.

Finding Probability Using a Table

One way to solve our problem is to set up a table that shows all of the possible outcomes, such as the one shown below.

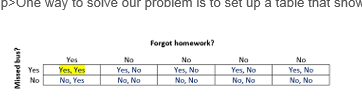
Using a table to show the possible outcomes

Since Gary misses the bus half of the time, we can show this with just two choices, YES and NO. The list across the top represents each of the five days that he goes to class. He forgets his homework one time out of the five days each week, so we represent this by showing YES one time, and NO for the other four times. The boxes in the table are completed to show the possible outcomes of whether Gary misses the bus and forgets his homework.

Out of the 10 possible outcomes, only one of them has a result of YES for both events. This gives us a probability of 1/10 for the compound event.

Finding Probability Using a Tree Diagram

The next strategy involves a tree diagram.

Using a tree diagram to show the possible outcomes

Each row lists the possibilities of that event happening. For our example, the first row shows the possibility of Gary forgetting his homework for each of the five days. Just as in the table, there is a YES for one day and No for the other four days. Beneath each day, we list the possibilities of him missing the bus for each of those days. There is a 50% chance that he will miss the bus, so we list one YES and one NO to show the possibility of him not missing the bus. Following each line of the diagram shows the different possible outcomes. Just as with the table, there are ten possible outcomes, of which one has yes for both events.

Finding Probability Using a Formula

These first two methods are simple and can help you to visualize the solution. However, it is not practical when you are working with large numbers or several events. For more complex situations, it is useful to use the following formula:

P(A, B) = P(A) x P(B)

Once we know the probability of each individual event, we multiply them together. This gives us the probability of both events happening at the same time. Even if we have more than two events, we just multiply all of their probabilities together.

Let's see how the formula would work for our example.

P(missing the bus, forgetting homework) = P(missing the bus) x P(forgetting homework) = 0.50 x 0.20 = 0.10

Gary has a 10% chance of missing the bus and forgetting his homework on the same day.

Lesson Summary

A compound event in math involves finding the probability of more than one event occurring at the same time. Drawing tables and diagrams are useful strategies when there are only a few events and a small number of outcomes. The formula P(A,B) = P(A) x P(B) can be used for any number of events and outcomes.