Lesson: Chapter - 17

Explanations

1. B

Simple harmonic motion is defined as the oscillation of an object about an equilibrium position where the restoring force acting on the object is directly proportional to its displacement from the equilibrium position.

Though we often treat pendulum motion as simple harmonic motion, this is in fact a simplification. The restoring force acting on a pendulum is mg sin?, where ? is the angle of displacement from the equilibrium position. The restoring force, then, is directly proportional to sin?, and not to the pendulum bob’s displacement, ?. At small angles, ? = sin?, so we can approximate the motion of a pendulum as simple harmonic motion, but the truth is more complicated.

The motion of a mass attached to a spring is given by Hooke’s Law, F = –kx. Since the restoring force, F, is directly proportional to the mass’s displacement, x, a mass on a spring does indeed exhibit simple harmonic motion.

There are two forces acting on a bouncy ball: the constant downward force of mg, and the occasional elastic force that sends the ball back into the air. Neither of these forces is proportional to the ball’s displacement from any point, so, despite the fact that a bouncy ball oscillates up and down, it does not exhibit simple harmonic motion.

Of the three examples given above, only a mass on a spring exhibits simple harmonic motion, so the correct answer is B.

2. B

The frequency, speed, and wavelength of a wave are related by the formula v = ?f. Solving for ?, we find:

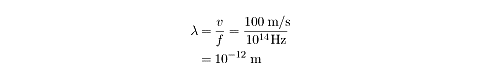
3. B

The speed v of a wave traveling along a string of mass m, length l, and tension T is given by: . This formula comes from the relationship between v, T, and string density m (namely, 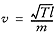) combined with the fact that density M = m/l. Since velocity is inversely proportional to the square root of the mass, waves on a string of quadrupled mass will be traveling half as fast.

4. D

The frequency of the beats produced by two dissonant sounds is simply the difference between the two frequencies. In this case, the piano tuner will hear beats with a frequency of 250 Hz – 200 Hz = 50 Hz.

5. B

A tube closed at one end can support a standing wave with a node at the closed end and an antinode at the open end. A tube open at both ends can support a standing wave with antinodes at both ends.

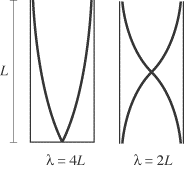
As the figure shows, the wavelength for a standing wave in a tube closed at one end is twice the wavelength for a standing wave in a tube open at both ends. Since frequency is inversely proportional to wavelength, the frequency for a standing wave in a tube closed at one end is half the frequency of a standing wave in a tube open at both ends.

6. E

When two waves move toward one another, they pass through each other without one affecting the other. While both waves are in the same place, they will superimpose to form a single wave that is the sum of the two waves, but once they have passed one another, they will continue on their trajectory unaffected.

7. A

The easiest way to solve this problem is through simple intuition. When you tighten a string, it plays at a higher pitch, and when you loosen a string, it plays at a lower pitch. Pitch and frequency are the same thing, so in order to raise the pitch of the piano string, the tuner has to tighten the string, thereby raising its fundamental frequency.

8. A

In general, the frequency heard by the person is given by the formula:

where ƒ_d and v_d are the frequency heard by the person and the velocity of the person, respectively, and ƒ_s and v_s are the frequency and the velocity of the police siren, respectively. Since the police car is traveling toward the person, the person will hear a higher frequency than that which the siren actually produces, so ƒ_d > ƒ_s. We also know that v_d = 0. If ƒ_d > ƒ_s, then the fraction in the equation above must be greater than one, so the denominator should read v – v_s, and not v + v_s. The resulting formula is:

9. C

Wavelength is related to velocity and frequency by the formula ? = v/f. In the previous question, we determined the frequency produced by the siren, so we can simply plug this formula into the formula for wavelength:

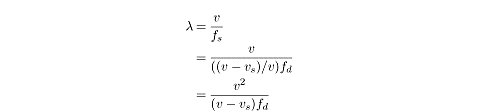
10. D

Generally speaking, the frequency heard by an observer is the frequency emitted at the source, multiplied by a factor of (v_s - v₀)/(v_s - v), where v_s is the speed of sound, v₀ is the velocity of the observer, and v is the velocity of the source of the sound. Solving for ƒ₀, the frequency heard by the observer, is just a matter of plugging the appropriate numbers into the formula:

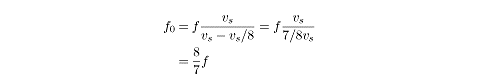
Common intuition should save you from answering A, B, or C: when an ambulance moves toward you, its siren sounds higher than it actually is.

Next to display next topic in the chapter.