The procedure for analyzing a collision depends on whether the process is
elastic or inelastic. Kinetic energy is conserved in elastic
collisions, whereas kinetic energy is converted into other forms of energy
during an inelastic collision. In both types of collisions, momentum is
conserved.
div>
Elastic Collisions
Anyone who plays pool has observed elastic collisions. In fact, perhaps you’d
better head over to the pool hall right now and start studying! Some kinetic
energy is converted into sound energy when pool balls collide—otherwise, the
collision would be silent—and a very small amount of kinetic energy is lost to
friction. However, the dissipated energy is such a small fraction of the ball’s
kinetic energy that we can treat the collision as elastic.
Equations for Kinetic Energy and Linear Momentum
Let’s examine an elastic collision between two particles of mass
m1
and
m2,
respectively. Assume that the collision is head-on, so we are dealing with only
one dimension—you are unlikely to find two-dimensional collisions of any
complexity on subject test Physics. The velocities of the particles before the elastic
collision are
V1
and
V2,
respectively. The velocities of the particles after the elastic collision are
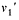and
.
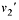Applying the law of conservation of linear momentum:
These two equations put together will help you solve any problem involving
elastic collisions. Usually, you will be given quantities for
m1,
m2,
v1
and
v2,
and can then manipulate the two equations to solve for
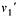and
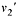
Example
A pool player hits the eight ball, which is initially at rest, head-on with the
cue ball. Both of these balls have the same mass, and the velocity of the cue
ball is initially
v1.
What are the velocities of the two balls after the collision? Assume the
Substituting
m1 = m2 = m
and
v2 = 0
into the equation for conservation of kinetic energy we find:
Applying the same substitutions to the equation for conservation of momentum, we
find:
If we square this second equation, we get:
By subtracting the equation for kinetic energy from this equation, we get:
The only way to account for this result is to conclude that
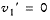and consequently
.
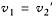In plain English, the cue ball and the eight ball swap velocities: after the
balls collide, the cue ball stops and the eight ball shoots forward with the
initial velocity of the cue ball. This is the simplest form of an elastic
collision, and also the most likely to be tested on subject test Physics.
Inelastic Collisionss
Most collisions are inelastic because kinetic energy is transferred to other
forms of energy—such as thermal energy, potential energy, and sound—during the
collision process. If you are asked to determine if a collision is elastic or
inelastic, calculate the kinetic energy of the bodies before and after the
collision. If kinetic energy is not conserved, then the collision is inelastic.
Momentum is conserved in all inelastic collisions.
On the whole, inelastic collisions will only appear on subject test Physics
qualitatively. You may be asked to identify a collision as inelastic, but you
won’t be expected to calculate the resulting velocities of the objects involved
in the collision. The one exception to this rule is in the case of completely
inelastic collisions.
Completely Inelastic Collisions
A completely inelastic collision, also called a “perfectly” or “totally”
inelastic collision, is one in which the colliding objects stick together upon
impact. As a result, the velocity of the two colliding objects is the same after
they collide. Because
,
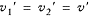it is possible to solve problems asking about the resulting velocities of
objects in a completely inelastic collision using only the law of conservation
of momentum.
Example
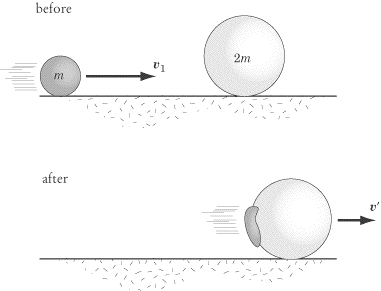
Two gumballs, of mass m and mass 2m respectively, collide head-on.
Before impact, the gumball of mass m is moving with a velocity
v1,
and the gumball of mass 2mm is stationary. What is the final velocity,
,
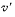of the gumball wad?
First, note that the gumball wad has a mass of
m + 2m = 3 m. The law of conservation of momentum
tells us that
,
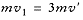and so
.
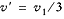Therefore, the final gumball wad moves in the same direction as the first
gumball, but with one-third of its velocity.
Collisions in Two Dimensionss
Two-dimensional collisions, while a little more involved than the
one-dimensional examples we’ve looked at so far, can be treated in exactly the
same way as their one-dimensional counterparts. Momentum is still conserved, as
is kinetic energy in the case of elastic collisions. The significant difference
is that you will have to break the trajectories of objects down into x-
and y-components. You will then be able to deal with the two components
separately: momentum is conserved in the x direction, and momentum is
conserved in the y direction. Solving a problem of two-dimensional
collision is effectively the same thing as solving two problems of
one-dimensional collision.
Because subject test Physics generally steers clear of making you do too much math,
it’s unlikely that you’ll be faced with a problem where you need to calculate
the final velocities of two objects that collide two-dimensionally. However,
questions that test your understanding of two-dimensional collisions
qualitatively are perfectly fair game.
Example
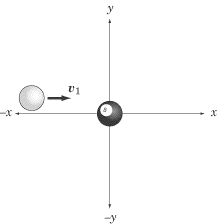
A pool player hits the eight ball with the cue ball, as illustrated above. Both
of the billiard balls have the same mass, and the eight ball is initially at
rest. Which of the figures below illustrates a possible trajectory of the balls,
given that the collision is elastic and both balls move at the same speed?
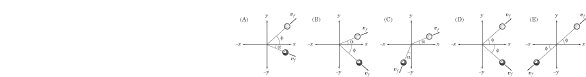
The correct answer choice is D, because momentum is not conserved in any
of the other figures. Note that the initial momentum in the y direction
is zero, so the momentum of the balls in the y direction after the
collision must also be zero. This is only true for choices D and E.
We also know that the initial momentum in the x direction is positive, so
the final momentum in the x direction must also be positive, which is not
true for E.
Back
Next
Next to display next topic in the chapter.
Practice Questions
Video Lessons and 10 Fully Explained Grand Tests
Large number of solved practice MCQ with explanations. Video Lessons and 10 Fully explained Grand/Full Tests.