2. D
Frequency and angular velocity are related by the formula
2pf,
and angular velocity and angular acceleration are related by the formula
a = d?dt.
In order to calculate the washing machine’s acceleration, then, we must
calculate its angular velocity, and divide that number by the amount of time it
takes to reach that velocity:
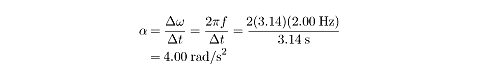
3. B
You need to apply the right-hand rule in order to solve this problem. Extend the
fingers of your right hand upward so that they point to the
0-second point on the clock face, and then
curl them around so that they point downward to the
30-second point on the clock face. In order
to do this, you’ll find that your thumb must be pointing inward toward the clock
face. This is the direction of the angular velocity vector.
4. D
The torque on an object is given by the formula t = r × F,
where F is the applied force
and r is the distance of the
applied force from the axis of rotation. In order to maximize this cross
product, we need to maximize the two quantities and insure that they are
perpendicular to one another. Statement I maximizes
F and statement III demands
that F and
r be perpendicular, but
statement II minimizes r
rather than maximizes it, so statement II is false.
5. C
The torque acting on the pendulum is the product of the force acting
perpendicular to the radius of the pendulum and the radius,
t=F1R .
A free-body diagram of the pendulum shows us that the force acting perpendicular
to the radius is
F1=mg sin?.
Since torque is the product of F1
and R, the torque is
mgR sin?.
6. D
The seesaw is in equilibrium when the net torque acting on it is zero. Since
both objects are exerting a force perpendicular to the seesaw, the torque is
equal to
.
The 3 kg mass exerts a torque of
N · m in the clockwise direction. The second mass exerts a torque in the
counterclockwise direction. If we know this torque also has a magnitude of 30g N
· m, we can solve for m:
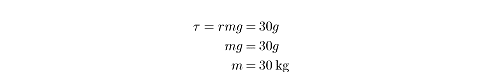
7. E
The rotational equivalent of Newton’s Second Law states that
.
We are told that
N · m and I =
1/2 MR2,
so now we can solve for
a:
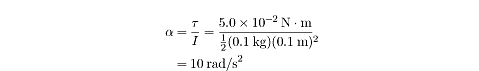
8. B
At the top of the incline, the disk has no kinetic energy, and a gravitational
potential energy of mgh. At the
bottom of the incline, all this gravitational potential energy has been
converted into kinetic energy. However, in rolling down the hill, only some of
this potential energy becomes translational kinetic energy, and the rest becomes
rotational kinetic energy. Translational kinetic energy is given by
1 /2
mv2 and rotational kinetic energy is given by
1 /2 I
?2.
We can express
?
in terms of v and
R with the equation
?
= v/R, and in the question we were
told that I =
1/2 mR2. We now have all
the information we need to solve for v:
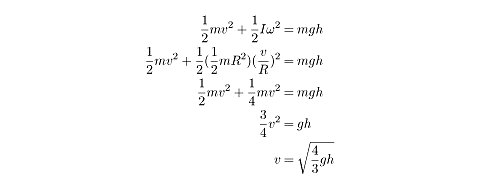
9. B
This is a conservation of momentum question. The angular momentum of the rock as
it is launched is equal to its momentum after it’s been launched. The momentum
of the rock-basket system as it swings around is:
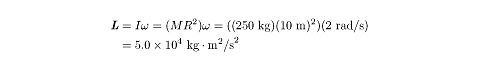
The rock will have the same momentum as it leaves the basket. The angular
momentum of a single particle is given by the formula
L = mvr. Since
L is conserved, we can manipulate
this formula and solve for v:
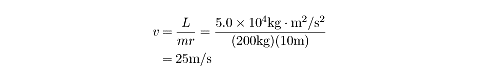
Be sure to remember that the initial mass of the basket-rock system is
250 kg, while the final mass of the rock is
only 200 kg.
10. C
Angular momentum,
L = l?,
is a conserved quantity, meaning that the greater
I is, the less
?
will be, and vice versa. In order to maximize angular velocity, then, it is
necessary to minimize the moment of inertia. Since the moment of inertia is
greater the farther the mass of a body is from its axis of rotation, we can
maximize angular velocity by concentrating all the mass near the axis of
rotation.
Next to display next topic in the chapter.
Practice Questions
Video Lessons and 10 Fully Explained Grand Tests
Large number of solved practice MCQ with explanations. Video Lessons and 10 Fully explained Grand/Full Tests.