Lesson: Chapter - 10

Important Definitions

There are a few basic physical concepts that are fundamental to a proper understanding of rotational motion. With a steady grasp of these concepts, you should encounter no major difficulties in making the transition between the mechanics of translational motion and of rotational motion.

Rigid Bodies

The questions on rotational motion on
Physics deal only with rigid bodies. A rigid body is an object that retains its overall shape, meaning that the particles that make up the rigid body stay in the same position relative to one another. A pool ball is one example of a rigid body since the shape of the ball is constant as it rolls and spins. A wheel, a record, and a top are other examples of rigid bodies that commonly appear in questions involving rotational motion. By contrast, a slinky is not a rigid body, because its coils expand, contract, and bend, so that its motion would be considerably more difficult to predict if you were to spin it about.

Center of Mass

The center of mass of an object, in case you have forgotten, is the point about which all the matter in the object is evenly distributed. A net force acting on the object will accelerate it in just the same way as if all the mass of the object were concentrated in its center of mass. We looked at the concept of center of mass in the previous chapter’s discussion of linear momentum. The concept of center of mass will play an even more central role in this chapter, as rotational motion is essentially defined as the rotation of a body about its center of mass.

Video Lesson - Center of Mass

Axis of Rotation

The rotational motion of a rigid body occurs when every point in the body moves in a circular path around a line called the axis of rotation, which cuts through the center of mass. One familiar example of rotational motion is that of a spinning wheel. In the figure at right, we see a wheel rotating counterclockwise around an axis labeled O that is perpendicular to the page.

As the wheel rotates, every point in the rigid body makes a circle around the axis of rotation, O.

Radians

We’re all very used to measuring angles in degrees, and know perfectly well that there are 360º in a circle, 90º in a right angle, and so on. You’ve probably noticed that 360 is also a convenient number because so many other numbers divide into it. However, this is a totally arbitrary system that has its origins in the Ancient Egyptian calendar which was based on a 360-day year.

Video Lesson - Arc Length

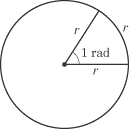
It makes far more mathematical sense to measure angles in radians (rad). If we were to measure the arc of a circle that has the same length as the radius of that circle, then one radian would be the angle made by two radii drawn to either end of the arc.

Converting between Degrees and Radians

It is unlikely that Physics will specifically ask you to convert between degrees and radians, but it will save you time and headaches if you can make this conversion quickly and easily. Just remember this formula:

Video Lesson - Degree to Radians

You’ll quickly get used to working in radians, but below is a conversion table for the more commonly occurring angles.

Calculating the Length of an Arc

The advantage of using radians instead of degrees, as will quickly become apparent, is that the radian is based on the nature of angles and circles themselves, rather than on the arbitrary fact of how long it takes our Earth to circle the sun.

Video Lesson - Finding Arc Length

For example, calculating the length of any arc in a circle is much easier with radians than with degrees. We know that the circumference of a circle is given by P = 2pr, and we know that there are 2p radians in a circle. If we wanted to know the length, l, of the arc described by any angle ?, we would know that this arc is a fraction of the perimeter, ( ?/2p)P. Because P = 2pr, the length of the arc would be:

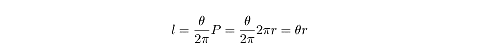
Next to display next topic in the chapter.