How Do I Use a System of Equations?

How Do I Use a System of Equations?

There are a few classic algebra word problems, such as the one about two trains traveling at different speeds. In this lesson, you'll learn how to take a word problem and convert it into the system of equations that will allow you to find the answer using either substitution or elimination.

Building a System of Equations

Probably the best known algebra word problem (which often seems to have been a traumatic experience for people) has to do with two trains leaving the station going opposite directions. It usually asks something along the lines of how fast they were going or how far apart they were. But I like to make math as un-traumatic as possible, so we're going to stay away from any train problems and instead, we're going to look at a difference classic word problem.

Let's say you're hosting a Super Bowl party and have been given $68 by all your friends that are coming to spend on pizza. You're going to get either cheese pizza or pepperoni pizza. Cheese pizzas cost $8 each, and the pepperoni pizzas cost $11 each. Since you might as well spend all the money (it's your friends', not yours) and you've also decided that 7 pizzas is the perfect amount of food, the question is how many of the seven pizzas should be cheese, and how many of them should be pepperoni?

We could try to guess and check our way through this problem, which might work for this one, but that's probably not going to work for most problems like this. So we'd rather use this example as a chance to learn how to do it with math. But in order to do it with math, we have to first change the words into mathematical equations. This can oftentimes be the hardest part.

In order to set up those equations, we need to figure out what the variables we're looking for are. In order to that, we're going to have to go back to the problem and figure out what it's really asking us for. This problem is asking us for how many cheese pizzas and how many pepperoni pizzas should we buy. Which means our variables should be the number of cheese pizzas (C) and the number of pepperoni pizzas (P). When we solve for those two things, we'll know how many we should buy.

So now that we have our variables, we have to come up with equations that represent the situation. This problem is going to need two equations to solve it because there are two variables, which means that this is a system of equations, because there's going to be more than one.

The easier equation to come up with uses the fact that we know we have to buy a total of 7 pizzas. We've decided that 7 pizzas is the perfect amount. Since C is the number of cheese and P is the number of pepperoni, and we know that the total has to be 7, my first equation is the number of cheese (C) plus the number of pepperoni (P) must equal 7: C + P = 7.

The harder equation to come up with has to do with the price and how much money we have and how much money different pizzas cost. What I want to do is set up an equation that equals 68. It should say that we are going to spend $68. This means I have to figure out how much money I'm spending on my cheese pizzas and how much money I'm spending on my pepperoni pizzas.

Using the substitution method to solve for C

Well, if we just think about this logically, if I bought one cheese pizza, it would cost me $8. And if I bought two, it would cost me $16. If I bought three, it would cost me $24, and so on. This means that the total money I spend on my cheese pizzas is simply 8 times the number of cheese pizzas I buy (8C). A very similar expression could be derived for the amount of money you spend on pepperoni pizzas by doing 11 times the number of pepperoni pizzas you buy (11P).

So 8C represents the money you spend on cheese pizzas and 11P represents the money you spend on pepperoni pizzas. If you add those two amounts together, that's the total amount we spend. And that has to be $68. So our second equation, the little more complicated one, is 8C + 11P = $68.

Solving a System of Equations with Substitution

Now that we have the system set up, we can actually begin to solve it. We're going to go over 2 ways of solving a system of equations, the first of which is called substitution. It is called this because we're going to take one expression from one equation and substitute it in for one of the variables in our second equation.

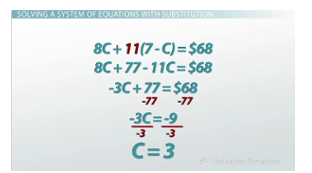
For example, we can take the equation that says C + P = 7, telling us the total number of pizzas we're getting, and we can solve this equation for P by undoing C from both sides with subtraction. We find an equivalent equation that says that P = 7 - C.

Now that I know what P equals, and since I want these two equations to be the same, I can substitute into the second equation what I now know what

Setting up equations to use the elimination method

I can use inverse operations to get the C by itself. First, you distribute the 11 to both terms: 8C + 77 - 11C = $68. Then, you can combine like terms, grouping the Cs together because they're on the same side of the equals sign: -3C + 77 = $68. Next, you undo an addition of 77 with a subtraction of 77: -3C = -9. Last but not least, you undo a multiplication of -3 with a division of -3 to both sides. You find that C, the number of cheese pizzas we're going to get, is 3: C = 3.

Now that I know the number of cheese pizzas, it's a pretty easy process to just take that and substitute back into the first equation: P = 7 - C. I now know that C is 3, which means that P = 7 - 3. This means that P is 4. I now know how many pepperoni pizzas we should buy.

Solving a System of Equations with Elimination

So that's the substitution method to solving a system of equations. But there's another method called the elimination method, which is really nice to use when both of my equations are in standard form. This means that both of my equations have the C's and the P's on the same side.

The elimination method requires us to add the two equations together in order to eliminate one of the variables. Right now, if I add these two equations together, none of my variables are going to eliminate. I'm going to end up with 9C and 12P and that doesn't really get me anywhere

But if I first multiply the top equation by -8, and I have to distribute it into everything, it changes that equation into: -8C - 8P = -56. If I leave the bottom equation the same and now add the two equations, my C's cancel. What I've done is that I've made the coefficients (which is a fancy word for the numbers in front of the variables) on my C variable opposites.

The 8 and the -8 cancel. I just get 0C. On the P's, I get -8P plus 11 P which is 3P. On the other side of the equals sign, I get -56 plus 68, which is 12: 0C + 3P = 12.

Solving for P using the elimination method

Now I have one equation with one variable. I can solve pretty quickly by undoing times 3 by dividing by 3, and we find that P = 4. The number of pepperoni pizzas is 4. Again, the same answer.

Once we have one answer, we do the same step as we did in the substitution method. We take our one answer and we substitute it back into one of the original equations. We now know that P = 4, so I know that C + 4 = 7. I undo adding 4 by subtracting 4 and I find that C = 3. We need 3 cheese pizzas.

Lesson Review

To review, step one to solving a word problem is to identify what the variables are by checking: what is the problem asking for?

Once you know what you want your variables to be, you need to set up the equations. Is there one variable? Then there's going to be one equation. Are there two variables? Then there are going to be two equations, and so on.

Step three, the last step, is to actually solve the problem using the substitution method or the elimination method. Substitution is nice when one equation is already solved for a variable. For example, when one of the equations already says x = or y = or c =. It requires you to take that one expression and substitute it in to the other equation for that same expression, then solve for the variables using inverse operations.

Elimination is nice when neither equation is solved for a variable, which means that both equations are in standard form (the x's and the y's are on the same side). It is then your job to make either pair of coefficients opposite numbers by multiplying one or both equations by something that will turn the coefficients into opposites. Once they're opposites, you can add the equations together, in order to eliminate one of the variables. Then, again, solve for the remaining variable using inverse operations and substitute it back in to find the second one.