Trigonometry: Sine and Cosine

Trigonometry: Sine and Cosine

Learn an easy trick to help you solve trigonometry problems, including problems with sine, cosine and inverse trig functions. At the end of this lesson, you'll know what SohCahToa means and how to use it.

Trigonometry Review

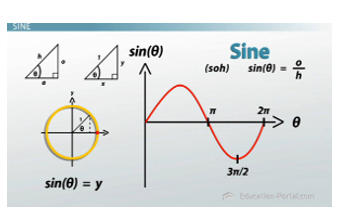
Graph of sine theta

Let's review some trigonometry. Let's say we have a unit circle - that is, a circle with a radius of 1 - and we're going to draw a point from the origin to the outside of the circle, some point x,y. The angle made between that line (between the origin and x,y) and the x-axis I'll call theta. What we're doing is basically taking a slice of pie out of our unit circle. Let's focus on that piece we just cut out. In particular, let's focus on the triangle made up of the radius 1, the x coordinate (which is one of the legs of this triangle) and the ycoordinate (the other leg of this triangle). That makes a right triangle. By the Pythagorean Theorem I can then say that x^2 + y^2 = 1 - that the sum of the square of both sides equals the square of the hypotenuse.

A Handy Mnemonic

Let's take another look at this right triangle, and let's define the edges. First I've got theta. Now theta is defined as the angle between our hypotenuse (which I'm going to call h) and this leg here (which I'm going to call a). That's because it's adjacent - next to - the angle. The other leg I'm going to call o; it's opposite the angle that I'm interested in. My triangle has two legs (a and o) and a hypotenuse (h). Why did I do that? Because I want to Saddle Our Horses, Canter Away Happily, To Other Adventures - SohCahToa. SohCahToa is a way to remember sines, cosines and tangents. Soh - 'saddle our horses' - means the sin of theta is equal to the opposite leg divided by the hypotenuse. Cah is for 'canter away happily' - cosine equals the adjacent over the hypotenuse. 'To other adventures' is the tangent of theta equals the opposite edge divided by the adjacent edge. If you're sneaky you can solve for tangent as a function of sine and cosine, and you find that tangent of theta equals sine of theta divided by the cosine of theta.

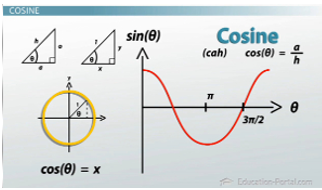
Graph of a cosine wave

Sine

So what does all this mean? Well, let's look at sine. Sine theta - Saddle Our Horses. Sine equals the opposite over the hypotenuse. If I go back to my unit circle, then my hypotenuse has a length of 1. My opposite edge has a length of y. So sine theta in the unit circle equals y. This helps us in graphing sine theta. So let's draw a graph - sine theta as a function of theta. What I'm going to do is go around the circle one time counterclockwise, and I'm going to watch what happens to y as theta goes from 0 all the way around to 2pi. 2pi is once around the circle. Then what happens to y? The y goes to -1 before going back to 0 as we complete the circle. So starting at pi, y goes to -1, then back up to 0 as we get to 2pi. So a graph of sine thetalooks like this. It's a pretty standard sine wave. It looks just like the ocean.

Cosine

Now let's look at cosine - Canter Away Happily. Cosine is equal to the adjacent edge divided by the hypotenuse. In our unit circle this is x divided by 1, or just x. I can do the same thing - what happens to x as I go around this circle counterclockwise? Initially x is equal to 1, then it slowly moves to 0, then -1, so we're tracing this out. When we're at 180 degrees, or pi, we're at x=-1, and then as we complete the circle we go back from -1 for x all the way up to 1 for x. So this is a cosine wave. It looks just like a sine wave, but it's shifted over by pi/2.

Graph of the tangent

Tangent

Lastly there's tangent. Tangent is a little bit harder. This is To Other Adventures. Tangent theta is equal to the opposite edge divided by the adjacent edge. It's harder because we aren't using 1 at all in this, we're using x and y, so tangent of theta in our unit circle is ydivided by x, or sine divided by cosine. When you plot out the tangent it starts out at 0, because when thetais 0, sine is 0 and cosine is 1, then it increases and eventually blows up to infinity - there's an asymptote at pi/2. Why do we have an asymptote at pi/2? Well, because cosine of pi/2 is 0, so we're dividing by 0 here, so here we've got our asymptote. Just past pi/2, tangent of theta is very negative, and then it increases, increases, increases… when we get to pi, tangent of pi is 0, then it follows this cycle again - it goes up to infinity, has a vertical asymptote at 3pi/2, and then comes back, minus infinity, back to 0, by the time we get to 2pi.

Okay, so we know SohCahToa - Saddle Our Horses, Canter Away Happily, To Other Adventures - hypotenuse, adjacent, opposite sides and our angle is theta. So sine theta equals opposite over hypotenuse, cosine theta is adjacent over hypotenuse and the tangent of theta is the opposite over the adjacent. But we also know that sometimes we don't care about the opposite over the hypotenuse; sometimes we care about the hypotenuse over the opposite. This is 1 divided by sine. 1 divided by sine is the cosecant. We just call that csc of theta. 1 divided by the cosine is the secant, sec, of theta. 1 divided by tangent is the cotangent of theta. In all of these, rather than using SohCahToa, we want to write out sine equals opposite over hypotenuse and take 1 divided by sine, 1 divided by opposite over hypotenuse. We end up with cosecant equals the hypotenuse over the opposite side, secant equals the hypotenuse over the adjacent side and the cotangent equals the adjacent divided by the opposite side.

A review of SohCahToa

Lesson Summary

So let's recap. If you remember nothing else from trigonometry, remember the Pythagorean Theorem. In this case you have a^2 + o^2 = h^2. And remember to Saddle Our Horses, Canter Away Happily, To Other Adventures.