
If we can find what terms must have been on the outside of this chart to get multiplied in and give us what we have here, we'll be done. We do this by dividing out the greatest common factor from each row and column of our chart. So, if I look at the top row of this chart, I have a 2x2 and -6x. I need to ask myself what do those things have in common? Well, 2 and 6 are both divisible by 2, so I can take out a 2. But they also both have an x, which means I also take out an x, so I can pull a 2x to the outside of that row. Going down a row to the bottom, 1x and -3, they don't have any factors with the numbers in common, and they also don't have any variables in common, which means the only thing I can divide out is a 1. Now, we go to the columns. Let's start in the left column, 2x2 and 1x. The numbers don't have anything in common but the variables do, which means I can take out 1x. Finally, the column on the right: -6x and -3. Both share a -3, which means I divide that out and write it on top. What we now have on the left and above our little area model is our factored answer. The terms that are on the same side are the terms that go in parentheses together to make up our two binomials, and I end up with (2x + 1)(x - 3). You can always quickly multiply out your answer to make sure you got the right thing, and if you do that here, looks like we're good.
Factoring quadratics like this take a while, and they're not always simple, so practice is key. Let's do one more example during this lesson.

Multiply the answer to make certain it is correct.
Another Example
Factor 9x2 - 4.
Not only is this example another one with a non-1 leading coefficient, it's also an example of a special quadratic that often messes students up. Why? Because there are no x's in the middle! It's not a trinomial! But that's okay. Just think of this problem as being this one: Factor 9x2 + 0x - 4. Now we're ready to go. We begin by finding the two numbers that fit the pattern. For this one, they'll have to add up to zero (the middle term) and multiply to -36, which was the 9 in front times the -4 on the end. Quickly looking through our options here and knowing that we're going to have to add up to zero makes it pretty obvious that 6 and -6 are going to be our winners. Now that we have these two values, we can fill in our area model. We put the 9x2and the -4 in one diagonal and the 6x and -6x in the other, but it actually doesn't matter which diagonal or even which order you put them in; they're all going to work! Lastly, we need to divide out the greatest common factors from each row and column to find out which binomials exist on the outside of this chart.
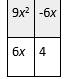
Looking at the top row, it looks like they both have a 3 and an x. The bottom row, both numbers are divisible by 2. The left column, I see a 3 and an x again, and the right column, they both have a -2 so I can pull that out. Rewriting the terms from each side together in parentheses as binomials says that the factored form of this is (3x + 2)(3x - 2).
Lesson Summary
Factoring problems with a leading coefficient that isn't 1 have two differences from their simpler counterparts. First, the pattern we use to determine the pair of numbers that will help us find our answer now requires you to find two numbers that have a product equal to the constant times the leading coefficient, instead of simply being the leading coefficient itself like before.
Secondly, once you come up with the pair of numbers that fit the pattern, you must substitute those numbers into an area model and factor out the greatest common factors to determine the answer. If you're asked to factor a quadratic that does not have an x term, just pretend that there is a 0x term in the middle there, and continue to do the problem just like you normally would.