Lesson: Data Sufficiency Challenging - 22t01

Unexpected Combinations: Example 1

[Page 22 of 24]

What is the probability that the length of AC is less than or equal to ?

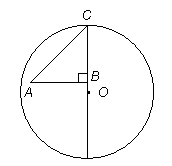
1) The area of circle O is 81.

2) The length of AB is 6.

This question deals with two different types of content - probability and geometry. To answer it, we must figure out whether we can determine a probability relationship, so let's start there.

The definition of probability appears below. Use it to determine what we must focus on to solve this question.

We want to know the probability that a certain line segment is equal to or less than a certain length. Well, to find any probability, we need to know the total number of possible outcomes and the number of desired outcomes (these being the number of ways to get the specific result we want). In this case, the number of desired outcomes will be all lengths of AC less than or equal to . The number of possible outcomes will be all possible lengths of AC. So basically we’re trying to figure out what fraction of all possible lengths of AC are less than or equal to . But what does the length of AC depend on? If AC had a fixed length, this question would make no sense, since the probability of its being less than or equal to would have to be either 100% or 0%. So the length of AC must depend on some other part of the diagram. But what? Now it’s time to look at the geometry.

Countinue

Back Next

Next to display next topic in the chapter.

Test Prep Lessons With Video Lessons and Explained MCQ

Quantitative Aptitude Reasoning English General Knowledge Picture Patterns

About us

EntryTest.com is a test preparation service for students seeking successful career.

About Disclaimer

Quick Test Prep

NUST NET IBA Karachi SAT LUMS LCAT LSE

 

NTS GAT NTS NAT GMAT GRE ACT

Study Abroad

Education Abroad Business Schools MS in USA