Lesson: Chapter - 4

Vector Addition

There are bound to be several questions on Physics test that involve vector addition, particularly in mechanics. The test doesn’t demand a very sophisticated understanding of vector addition, but it’s important that you grasp the principle. That is, you won’t be asked to make complicated calculations, but you will be expected to know what happens when you add two vectors together.

The easiest way to learn how vector addition works is to look at it graphically. There are two equivalent ways to add vectors graphically: the tip-to-tail (head to tail) method and the parallelogram method. Both will get you to the same result, but one or the other is more convenient depending on the circumstances.

Head to Tail Method

We can add any two vectors, A and B, by placing the tail of B so that it meets the tip of A. The sum, A + B, is the vector from the tail of A to the tip of B.

Note that you’ll get the same vector if you place the tip of B against the tail of A. In other words, A + B and B + A are equivalent.

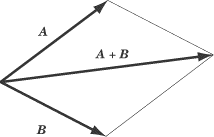
Parallelogram Method

To add A and B using the parallelogram method, place the tail of B so that it meets the tail of A. Take these two vectors to be the first two adjacent sides of a parallelogram, and draw in the remaining two sides. The vector sum, A + B, extends from the tails of A and B across the diagonal to the opposite corner of the parallelogram. If the vectors are perpendicular and unequal in magnitude, the parallelogram will be a rectangle. If the vectors are perpendicular and equal in magnitude, the parallelogram will be a square.

Adding Vector Magnitudes

Of course, knowing what the sum of two vectors looks like is often not enough. Sometimes you’ll need to know the magnitude of the resultant vector. This, of course, depends not only on the magnitude of the two vectors you’re adding, but also on the angle between the two vectors.

Adding Perpendicular Vectors

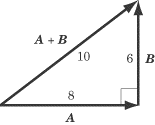
Suppose vector A has a magnitude of 8, and vector B is perpendicular to A with a magnitude of 6. What is the magnitude of A + B? Since vectors A and B are perpendicular, the triangle formed by A, B, and A + B is a right triangle. We can use the Pythagorean Theorem to calculate the magnitude of A + B, which is

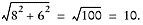
Adding Parallel Vectors

If the vectors you want to add are in the same direction, they can be added using simple arithmetic. For example, if you get in your car and drive eight miles east, stop for a break, and then drive six miles east, you will be 8 + 6 = 14 miles east of your origin. If you drive eight miles east and then six miles west, you will end up 8 – 6 = 2 miles east of your origin.

Adding Vectors at Other Angles

When A and B are neither perpendicular nor parallel, it is more difficult to calculate the magnitude of A + B because we can no longer use the Pythagorean Theorem. It is possible to calculate this sum using trigonometry, but subject test Physics will never ask you to do this. For the most part, subject test Physics will want you to show graphically what the sum will look like, following the tip-to-tail or parallelogram methods. On the rare occasions that you need to calculate the sum of vectors that are not perpendicular, you will be able to use the component method of vector addition, explained later in this chapter.

Example

Vector A has a magnitude of 9 and points due north, vector B has a magnitude of 3 and points due north, and vector C has a magnitude of 5 and points due west. What is the magnitude of the resultant vector, A + B + C?

First, add the two parallel vectors, A and B. Because they are parallel, this is a simple matter of straightforward addition: 9 + 3 = 12. So the vector A + B has a magnitude of 12 and points due north. Next, add A + B to C. These two vectors are perpendicular, so apply the Pythagorean Theorem:

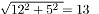
The sum of the three vectors has a magnitude of 13. Though a little more time-consuming, adding three vectors is just as simple as adding two.

Next to display next topic in the chapter.