Angular Frequency
Angular frequency, f, is
defined as the number of circular revolutions in a given time interval. It is
commonly measured in units of Hertz (Hz), where 1
Hz = 1 s–1. For example, the
second hand on a clock completes one revolution every
60 seconds and therefore has an angular
frequency of
1 /60Hz.
Video Lesson - Frequency
The relationship between frequency and angular velocity is:
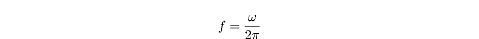
For example, the second hand of a clock has an angular velocity of
? = d?/dt = 2p/60
s. Plugging that value into the equation above, we get

which we already determined to be the frequency of the second hand of a clock.
Angular Period
Angular period, T, is defined
as the time required to complete one revolution and is related to frequency by
the equation:
T = 1/f
Since we know that the frequency of the second hand is
1/60 Hz, we can quickly see that the period of the
second hand is 60 s. It takes
60 seconds for the second hand to complete
a revolution, so the period of the second hand is
60 seconds. Period and angular velocity are related by the equation
T = 2p/?
Example
|
|
The Earth makes a complete rotation around the sun once every 365.25 days. What
is the Earth’s angular velocity? |
The question tells us that the Earth has a period of
T =
365.25 days. If we plug this value into the equation relating period and
angular velocity, we find:
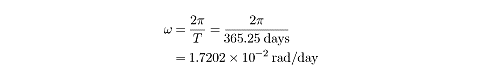
Note, however, that this equation only gives us the Earth’s angular velocity in
terms of radians per day. In terms of radians per second, the correct answer is:
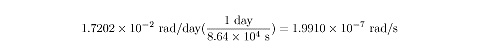
Relation of Angular Variables to Linear Variables
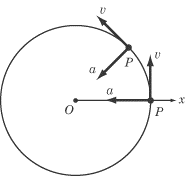
At any given moment, a rotating particle has an instantaneous linear velocity
and an instantaneous linear acceleration. For instance, a particle
P that is rotating counterclockwise
will have an instantaneous velocity in the positive
y direction at the moment it is at
the positive x-axis. In general, a rotating particle has an instantaneous
velocity that is tangent to the circle described by its rotation and an
instantaneous acceleration that points toward the center of the circle.
On Physics, you may be called upon to determine a particle’s linear
velocity or acceleration given its angular velocity or acceleration, or vice
versa. Let’s take a look at how this is done.
Distance
We saw earlier that the angular position, F,
of a rotating particle is related to the length of the arc,
l, between the particle’s present position and the positive x-axis by the equation
F = l/r, or l = F r. Similarly, for any angular displacement,
?, we can say that the length, l, of
the arc made by a particle undergoing that displacement is
l = ?r
Note that the length of the arc gives us a particle’s distance traveled rather
than its displacement, since displacement is a vector quantity measuring only
the straight-line distance between two points, and not the length of the route
traveled between those two points.
Velocity and Acceleration
Given the relationship we have determined between arc distance traveled,
l, and angular displacement, ?, we can now find expressions to relate linear and angular velocity and
acceleration.
We can express the instantaneous linear velocity of a rotating particle as
v = l/t, where
l is the distance traveled along the
arc. From this formula, we can derive a formula relating linear and angular
velocity:
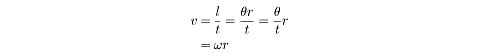
In turn, we can express linear acceleration as
a = v/t, giving us this formula relating linear and angular
acceleration:
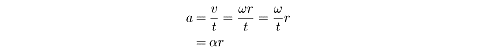
Example
The radius of the Earth is approximately
6.4 × 106
m. What is the instantaneous velocity of a point on the surface of the Earth at
the equator?
We know that the period of the Earth’s rotation is
24 hours, or
8.64 × 104
seconds. From the equation relating period, T,
to angular velocity,
?,
we can find the angular velocity of the Earth:

Now that we know the Earth’s angular velocity, we simply plug that value into
the equation for linear velocity:
v = wr = (7.27 ×10-5rad/s)(6.4 ×106m) = 4.7 ×2m/s
They may not notice it, but people living at the equator are moving faster than
the speed of sound.
Equations of Rotational Kinematics
In Chapter 2 we defined the kinematic equations for bodies moving at constant
acceleration. As we have seen, there are very clear rotational counterparts for
linear displacement, velocity, and acceleration, so we are able to develop an
analogous set of five equations for solving problems in rotational kinematics:
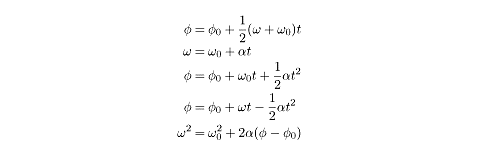
In these equations,
?0
is the object’s initial angular velocity at its initial position,
ø0.
Any questions on Physics that call upon your knowledge of the kinematic
equations will almost certainly be of the translational variety. However, it’s
worth noting just how deep the parallels between translational and rotational
kinematics run.
Vector Notation of Rotational Variables
Angular velocity and angular acceleration are vector quantities; the equations
above define their magnitudes but not their directions. Given that objects with
angular velocity or acceleration are moving in a circle, how do we determine the
direction of the vector? It may seem strange, but the direction of the vector
for angular velocity or acceleration is actually perpendicular to the plane in
which the object is rotating.
We determine the direction of the angular velocity vector using the
right-hand rule. Take your right hand and curl your fingers along the path
of the rotating particle or body. Your thumb then points in the direction of the
angular velocity of the body. Note that the angular velocity is along the body’s
axis of rotation.
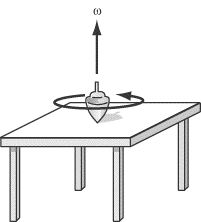
The figure below illustrates a top spinning counterclockwise on a table. The
right-hand rule shows that its angular velocity is in the upward direction. Note
that if the top were rotating clockwise, then its angular velocity would be in
the downward direction.
To find the direction of a rigid body’s angular acceleration, you must first
find the direction of the body’s angular velocity. Then, if the magnitude of the
angular velocity is increasing, the angular acceleration is in the same
direction as the angular velocity vector. On the other hand, if the magnitude of
the angular velocity is decreasing, then the angular acceleration points in the
direction opposite the angular velocity vector.
Next to display next topic in the chapter.
Practice Questions
Video Lessons and 10 Fully Explained Grand Tests
Large number of solved practice MCQ with explanations. Video Lessons and 10 Fully explained Grand/Full Tests.