Calculating Magnetic Force
A magnetic field exerts a force on a moving charge. Given a magnetic field,
B, and a charge,
q, moving with velocity,
v, the force,
F, on the charge is:
F = q(v × B)
Magnetic field strength is measured in teslas (T), where
1 T = 1
N/A · m.
Video Lesson
You’ll notice that the force on a moving particle is calculated as a cross
product of the particle’s velocity and the magnetic field’s strength. You can
determine the direction of the
v × B
vector by using the right-hand rule as follows: point the fingers of your right
hand in the direction of the velocity vector and then curl them around to point
in the direction of the magnetic field vector. The direction in which your thumb
points gives you the direction of the v × B vector.
However, though q is a scalar
quantity, it can affect the direction of the force vector. If
q has a negative value, then
q(v × B)
has a negative value, and so the force vector will point in a direction opposite
from what the right-hand rule might tell you.
You can calculate the magnitude of the magnetic force without using the
right-hand rule, so long as you know the angle, ?, between the velocity vector and the magnetic field vector:
F = qvBsin?
The sin
?
term is important, because it lets us see very quickly that there is no force if
a charge moves parallel to a magnetic field, and that the greatest force occurs
when a charge moves perpendicular to the magnetic field.
Example

A charge of 5 C moves upward at 2 m/s in a magnetic field of 0.3 T that points
into the page. What is the magnitude and direction of the force that the charge
experiences?
The cross product of
v × B
is a vector of magnitude qvB sin
sin?
= 3 N. Following the right-hand rule, point
your fingers toward the top of the page, and then curl them around so that they
point into the page. You’ll find that your thumb is pointing left, which is the
direction of the
v × B
vector. Because the value of q is
positive, the force acting on the particle will also be in the leftward
direction.
A Quick Note on Vectors Going In and Out of the Page
The magnetic field lines illustrated in this example that are going into the
page are represented by circles with an “x” inscribed in them. Vector lines
pointing out of the page are represented by circles with a dot in them. You can
think about these symbols as arrows appearing from in front or behind: from in
front, you see the conical tip of the arrow, and from behind you see the
fletching of the four feathers in an “x” shape.
Trajectory of Charges in a Magnetic Field
The direction of the force on a moving charge depends on the direction of its
velocity. As its velocity changes, so will its direction. The magnitude of
the velocity will not change, but charged particles moving in a magnetic
field experience nonlinear trajectories.
When the Velocity Vector and Magnetic Field Lines Are Perpendicular
In the example above, we saw that a force of 3
N would pull the charged particle to the left. However, as soon as the
particle begins to move, the velocity vector changes, and so must the force
acting on the particle. As long as the particle’s velocity vector is at a right
angle to the magnetic field lines, the force vector will be at right angles to
both the velocity vector and the magnetic field. As we saw in the chapter on
circular motion and gravitation, a force that always acts perpendicular to the
velocity of an object causes that object to move in circular motion.
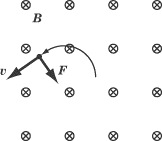
Because the velocity vector and the magnetic field lines are at right angles to
one another, the magnitude of the magnetic force is
F = qvB. Furthermore, because the
magnetic force pulls the particle in a circular path, it is a centripetal force
that fits the equation F = mv2/r.
Combining these two equations, we can solve for
r to determine the radius of the circle of the charged particle’s
orbit:

When the Velocity Vector and Magnetic Field Lines Are Parallel
The magnetic force acting on a moving charged particle is the cross product of
the velocity vector and the magnetic field vector, so when these two vectors are
parallel, the magnetic force acting on them is zero.
When the Velocity Vector and Magnetic Field Lines Are Neither Perpendicular nor
Parallel
The easiest way to deal with a velocity vector that is neither parallel nor
perpendicular to a magnetic field is to break it into components that are
perpendicular and parallel to the magnetic field.

The x-component of the velocity vector illustrated above will move with
circular motion. Applying the right-hand rule, we find that the force will be
directed downward into the page if the particle has a positive charge. The y-component
of the velocity vector will experience no magnetic force at all, because it is
moving parallel to the magnetic field lines. As a result, the charged particle
will move in a helix pattern, spiraling around while also moving up toward the
top of the page. Its trajectory will look something like this:
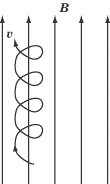
If the particle has a positive charge it will move in a counterclockwise
direction, and if it has a negative charge it will move in a clockwise
direction.
Example

A particle of mass
1 × 10-5
kg has a negative charge of –10 C. It moves in a clockwise circular pattern of
radius 2 m at a speed of
1 × 103
m/s. What is the magnitude and direction of the magnetic field acting upon it?
We know the velocity, mass, charge, and radius of the orbit of the particle.
These four quantities are related to magnetic field strength,
B, in the equation
r = mv/qB. By rearranging this
equation, we can solve for B:
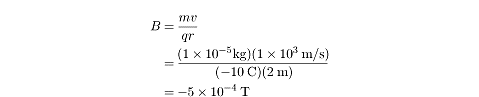
Now we just need to determine the direction of the magnetic field. To find the
direction, apply the right-hand rule in reverse: point your thumb in the
direction of the force—toward the center of the circle—and then stretch your
fingers in the direction of the velocity. When you curl your fingers around,
they will point out of the page. However, because the particle has a negative
charge, the magnetic field has the opposite direction—into the page.
Magnetic Fields and Electric Fields Overlapping
There’s no reason why a magnetic field and an electric field can’t operate in
the same place. Both will exert a force on a moving charge. Figuring out the
total force exerted on the charge is pretty straightforward: you simply add the
force exerted by the magnetic field to the force exerted by the electric field.
Let’s look at an example.
Example
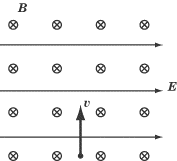
A particle with a positive charge of 3 C moves upward at a speed of 10 m/s. It
passes simultaneously through a magnetic field of 0.2 T directed into the page
and an electric field of 2 N/C directed to the right. How is the motion of the
particle affected?
Answering this question is a matter of calculating the force exerted by the
magnetic field and the force exerted by the electric field, and then adding them
together. The force exerted by the magnetic field is:
F =qvB = (3C)(10m/s) (0.2T) = 6N
Using the right-hand-rule, we find that this force is directed to the left. The
force exerted by the electric field is:
F = qE = (3C)(2N/C) = 6 N
This force is directed to the right. In sum, we have one force of
6 N pushing the particle to the left and
one force of 6 N pushing the particle to
the right. The net force on the particle is zero, so it continues toward the top
of the page with a constant velocity of 10
m/s.
Next to display next topic in the chapter.
Practice Questions
Video Lessons and 10 Fully Explained Grand Tests
Large number of solved practice MCQ with explanations. Video Lessons and 10 Fully explained Grand/Full Tests.