Solving Problems Using Rational Equations

Solving Problems Using Rational Equations

Follow the steps you will learn in this video lesson to help you solve rational equation problems. See how the process turns what looks like a huge problem into a much simpler and easier to manage problem.

Rational Equations

What is a rational equation? The name sounds worse than what it actually is. A rational equation is just an equation that has fractions. These fractions might just be numbers, but they also may be polynomials with numbers and letters in them. Think of the equations you work with in algebra when you are solving for a particular variable. A rational equation will look similar to those except that it will have a fraction on one or both sides of the equation.

What you see below is an example of a rational equation.

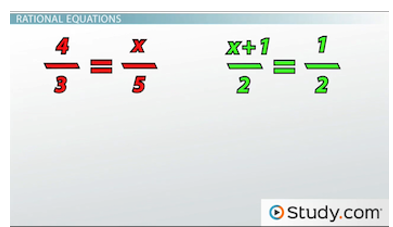
Examples of rational equations

Let me show you some more. All of the ones below are also rational equations.

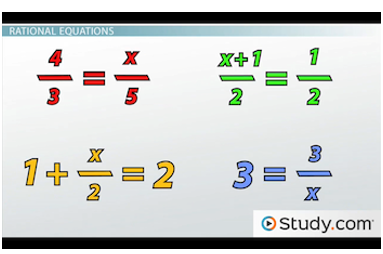
More examples of rational equations

A good way to recognize rational equations is to look for fractions. If you see a fraction made up of only numbers and/or variables, an equal sign and other numbers and/or variables, then you are looking at a rational equation.

The Problem

What I want to show you in this video lesson is a way to solve any kind of rational equation. I know there are shortcuts out there, and you will find them if you search. But, these shortcuts have limitations and can sometimes be only used on a particular type of equation. The process I want you to take home from this lesson is one that can be applied to all rational equations. It's not hard, and I will give you helpful tips on how to remember each step.

The problem we will use to go over this information is the very first one you saw in this lesson.

4 / 3 = x / 5

Real quick: how do you know that this is a rational equation? What is it that you are looking for to help you recognize it? That's right, fractions! And, this one happens to have one fraction on each side of the equals sign.

Dealing with the Denominator

Remember, fractions are not your enemy. Yes, I said that. It's true. But, don't worry - our very first step is to work at rewriting this equation without fractions. It can be done, and I will show you an easy way. Fractions are really not bad at all when you focus on the numerator and denominator separately.

This is exactly what we are going to do. We are going to focus on just the denominator. A good way to remember this first part of solving the problem is to think about what identifies a rational equation. Yes, fractions, and fractions have denominators.

Step 1 is to find the common denominator. I remember this step easily by thinking of the first step I usually take when adding or working with regular fractions. We always start by finding a common denominator. Because rational equations can involve variables, finding our common denominator is slightly different than when working with regular fractions. It's actually easier, I think. What you do is you look at the various denominators that you have, and all you need to do is make sure that your common denominator has all the denominators in it and multiplied together. Our problem has denominators of 3 and 5, so our common denominator will be 3 * 5. I'm going to leave it in the multiplication form because this will make my next step easier.

Step 2 is to multiply the whole problem by the common denominator. Remember this step by remembering how with regular fractions you want all the fractions to have the common denominator, but in our case, we will multiply everything by the common denominator. When we do this, we can cancel like terms, and we end up with an equation that doesn't have fractions.

(3 * 5) 4 / 3 = (3 * 5) x / 5

(5 * 4) = (3 * x)

20 = 3x

Freedom! Doesn't this equation look much easier to solve than the one we started with? You see, fractions really aren't all that bad.

Finding Our Solution

Step 3, now, is to solve the equation for our variable. You can remember this step by also thinking of regular fractions and what you do with them after you find the common denominator. You do the same and solve the problem, either getting the answer or solving a variable. We will continue to use our algebra skills to solve this problem. We will divide both sides by 3 to isolate our x variable. Once we have x by itself, we have found our answer.

20 = 3x

x = 20 / 3

It looks like our answer is 20 / 3. It might seem like we are done here, but we have one more step to do.

Step 4 is to check to make sure our answer does not produce a division by zero in our original problem. Remember this step by thinking of your teacher. You want to double-check your work so you can prove to your teacher that your answer is correct. To do this, we will look at our original problem, plug in our answer and see if it produces a division by zero anywhere. If it doesn't, then our answer is good to go. If it does, then our answer is not a valid one.

When your answer produces division by zero, it doesn't mean that you did something wrong, it just means that this particular problem is not defined at that point. It is possible to have an answer of 'no real solutions.' I see that it doesn't produce any division by zero, so that means my answer checks out. My final answer is 20 / 3.

Lesson Summary

In summary, rational equations are equations with fractions in them. The most important things I want you to take away from this video are the steps to solve them. There are only four of them, and they are similar to the ones you follow to solve regular fraction problems. Step 1 is to find the common denominator. Step 2 is to multiply everything by the common denominator. Step 3 is to solve for the variable. And, step 4 is to check the answer to make it sure it doesn't produce division by zero, which is undefined. Use the steps to working with regular fractions as a memory aid to help you remember these four steps. Fractions really are not your enemy.