Applying Scale Factors to Perimeter, Area, and Volume of Similar Figures

Applying Scale Factors to Perimeter, Area, and Volume of Similar Figures

How do shapes change sizes yet retain their proportions and similarities to other shapes? In this lesson, we'll look at what a scale factor is and how to apply it. We'll consider scale factors with regards to three different aspects of similar shapes: perimeter, area and volume.

Issues of Scale

When I was a kid, I had all kinds of action figures - He-Man, Star Wars, Ghostbusters. I liked to play with them together, but that didn't always make sense. One set of action figures in particular, Thunder cats, never fit. The Thunder cats action figures were way bigger than all the other action figures. So, a Thunder cat could never pilot a Star Wars speeder bike. And, my Han Solo figure was way too small for the Thunder cats' vehicles. The scales just weren't the same. This is an example of why scale factors matter.

What Is a Scale Factor?

A scale factor is simply a number that multiplies the dimensions of a shape. This can make a shape larger. Larger shapes will have a scale factor greater than one. So, if the scale factor is three, then the dimensions of the new shape will be three times larger than that of the original.

Let's say you own a doughnut shop, and you want a giant strawberry-frosted doughnut on top of your shop. You might use a scale factor of 25. Every tasty inch of a regular doughnut would be 25 inches on the model. So, a 5-inch doughnut would be 125 inches, or almost 10.5 feet tall.

A scale factor can also make a shape smaller. Smaller shapes will have a scale factor of less than one. You've probably seen this with Matchbox cars, which are often shrunk-down versions of real cars. This also works with dollhouses. For example, a model of a house may have a scale factor of 1/50. That means that 1 inch on the model is equal to 50 inches on the actual house. So, a 4-foot-tall dresser would be about 1-inch-tall in the model.

If the scale factor is one, then the two shapes are the same, or congruent. The scale factor of the car below to the other car is one. That's not very interesting, so we don't usually talk about scale factors of one.

These two cars are congruent.

If you're into variables, the equation for a scale factor can be written as y = Cu, where x is the original dimension, y is the new dimension, and C is the scale factor, or the amount by which the original is multiplied.

Perimeter Examples

Let's talk about how scale factors influence shapes in geometry, starting with perimeter.

Here's a rectangle:

This rectangle has a perimeter of 16.

Its width is 3 and its length is 5. What's the perimeter? 3 + 3 + 5 + 5, or 16. Now, let's apply a scale factor of 4 for the new rectangle. It will have dimensions that are 4 times that of the original. Instead of a width of 3, it will be 3 x 4, or 12. And, instead of a length of 5, it'll be 5 x 4, or 20. This new rectangle is similar to the original, which means it has the same shape, but not necessarily the same size.

The scale factor is equal to the perimeter change.

What impact did this have on the perimeter? The new perimeter is 12 + 12 + 20 + 20, or 64. 16 to 64? That's 4 times the original. So, the change in perimeter is equal to the scale factor.

Area Examples

Things are a little different with area. Let's look at those same two rectangles:

Squaring the scale factor leads to the change in area.

What's the area of the first? The area of a rectangle is length times width, so it's 5 x 3, which is 15. What about the second one? 20 x 12, which is 240. Does 15 x 4 = 240? No. What is the relationship between 15 and 240? If you divide 240 by 15, you get 16. And, what was our scale factor? 4. 16 is 4^2. So, the change in area is equal to the scale factor squared.

Let's look at another example. Here's a triangle with a base of 5 and a height of 4:

This triangle has an area of 10.

The area of a triangle is ½ times base times height. So, this triangle's area is ½ x 5 x 4, which is 10. Let's make a new triangle using a scale factor of 3. This new triangle has a base of 15 and a height of 12. Its area is ½ x 15 x 12, or 90.

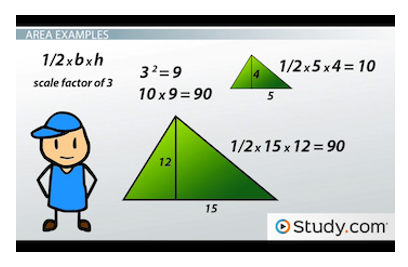
This triangle has an area of 90.

When you're working with scale factors, square the scale factor to determine the area of the new figure. If you think about it, it makes sense why area would be the scale factor squared. Area involves two dimensions multiplied together. With scale factor, all you're really doing is multiplying the scale factor times itself.

Volume Examples

Next, there's volume. Like going from perimeter to area, going from area to volume means adding a layer. In this case, it's a third dimension. Instead of squaring the scale factor, guess what? We're going to cube it! So, the change in volume is equal to the scale factor cubed. Cubing a number is raising it to the third power. So, if you remember that volume involves three dimensions, you can remember to cube the scale factor. Let's try this out.

Here's a rectangular prism:

The volume of this rectangular prism equals 24 cubic inches.

Let's make this interesting. Let's say it's a box of cookies. It's 4 inches by 2 inches by 3 inches. The volume of a rectangular prism will be length times width times height. So, its volume is 4 x 2 x 3, or 24 cubic inches. That's not going to hold a lot of cookies, even if they're small. So, let's scale it! Let's use a scale factor of 3.

Here's our new box:

This is the box of cookies scaled by three.

It's 12 by 6 by 9. The volume of this box will then be 12 x 6 x 9, which is 648 cubic inches. Now that box will hold a lot of cookies. We just need some milk.

Oh, but what about the change in volume? I said it's the scale factor cubed. But, what's 3 cubed? It's 27. And, what happens if we multiply the original volume, 24, times 27? Yep. It's 648.

But, what about that milk? Let's do one more volume example. Here's a kid's size glass of milk:

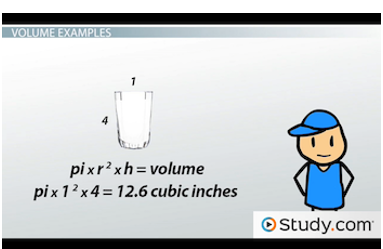
The volume of this glass of milk is 12.6 cubic inches.

The volume of a cylinder is pi times r^2 times h, where r is the radius of the circle on the top and h is the height. This glass has a radius of 1 inch and a height of 4 inches. So, its volume is pi x 1^2 x 4, or about 12.6 cubic inches.

We have a huge box of cookies, so we need a bigger glass of milk. What scale factor should we use? Since scale factors are cubed with volume, remember that even a small change will have significant ramifications.

Let's try a scale factor of 2. That will make our radius 2 inches and our height 8 inches. That doesn't seem unreasonable, right? Now, we don't need to do the volume formula. We can just cube the scale factor. 2^3 = 8. If our original volume was 12.6, then our new volume is 12.6 x 8, or 101 cubic inches. For those of you who don't think of your milk in cubic inches, that's about 1.75 quarts of milk. Holy cow!

Lesson Summary

In summary, a scale factor is simply a number that multiplies the dimensions of a shape. It's a way of describing the relationship between similar shapes, whether it's a house and a dollhouse-size replica or a pair of triangles.

The perimeter of a scaled object will be equal to the scale factor. If the scale factor is three, then the perimeter of the new object will be three times the original perimeter. The area of a scaled object will be equal to the scale factor squared. Again, if the scale factor is three, the area of the new object will be nine times, or three squared, the area of the original object. Finally, the volume of a scaled object will be equal to the scale factor cubed. So, if the scale factor is three, the volume of the new object will be three cubed, or 27 times, the volume of the original object.