Electron Configurations

Using the periodic table, determine the electron configuration for sulfur.

Explanation

First locate sulfur in the periodic table; it is in the third period, in the p block of elements. Count from left to right in the p block, and you determine that sulfur’s valence electrons have an ending configuration of 3p⁴, which means everything up to that sublevel is also full, so its electron configuration is 1s²2s²2p⁶3s²3p⁴. You can check your answer—the neutral sulfur atom has 16 protons, and 16 electrons. Add up the number of electrons in your answer: 2 + 2 + 6 + 2 + 4 = 16.

Another way of expressing this and other electron configurations is to use the symbol for the noble gas preceding the element in question, which assumes its electron configuration, and add on the additional orbitals. So sulfur, our example above, can be written [Ne] 3s²3p⁴.

Orbital notation is basically just another way of expressing the electron configuration of an atom. It is very useful in determining quantum numbers as well as electron pairing. The orbital notation for sulfur would be represented as follows:

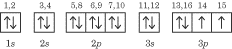
Notice that electrons 5, 6, and 7 went into their own orbitals before electrons 8, 9, and 10 entered, forcing pairings in the 2p sublevel; the same thing happens in the 3p level.

Now we can determine the set of quantum numbers. First, n = 3, since the valence electron (the outermost electron) is a 3p electron. Next, we know that p sublevels have an l value of 1. We know that ml can have a value between l and -l, and to get the ml quantum number, we go back to the orbital notation for the valence electron and focus on the 3p sublevel alone. It looks like this:

Simply number the blanks with a zero assigned to the center blank, with negative numbers to the left and positive to the right of the zero. The last electron was number 16 and “landed” in the first blank as a down arrow, which means its m_l = -1 and m_s = -1/2, since the electron is the second to be placed in the orbital and therefore must have a negative spin.

So, when determining m_l, just make a number line underneath the sublevel, with zero in the middle, negative numbers to the left, and positive numbers to the right. Make as many blanks as there are orbitals for a given sublevel. For assigning m_s, the first electron placed in an orbital (the up arrow) gets the +1/2 and the second one (the down arrow) gets the -1/2.

Which element has this set of quantum numbers: n = 5, l = 1, ml = -1, and ms = -1/2?

Explanation

First, think about the electron configuration: n = 5 and l = 1, so it must be a 5p electron. The ms quantum number corresponds to this orbital notation picture:

Be sure to number the blanks and realize that the -1/2 means it is a pairing electron! The element has a configuration of 5p⁴; so it must be tellurium.

Example

Complete the following table:

Element	Valence electron configuration	Valence orbital notation	Set of quantum numbers
	[Ar] 3d6
			5, 1, 0, +1/2
	4p5
			6, 0, 0, -1/2

Answer: element	Valence electron configuration	Valence orbital notation	Set of quantum number (n, l, ml, ms)s
K	[Ar] 4s1		4, 0, 0, +1/2
Fe	[Ar] 4s2 3d 6		3, 2, -2, -1/2
N	<1s22s 22p 3/td>		2, 1, 1, +1/2
Sn	[Kr] 5s24d105p2		5, 1, 0, +1/2
Br	[Ar] 4s 2 3d104p5		4, 1, 0, -1/2
Ba	[Xe] 6s2		6, 0, 0, - 1/2