1. Sum of the Interior Angles
If you were trapped on a desert island and had to take the Subject Math test, this is
the one rule about triangles you should bring along: the sum of the measures of
the interior angles is 180º. With this rule, if you know the measures of two of
a triangle’s angles, you will be able to find the third. Helpful, don’t you
think?

2. Measure of an Exterior Angle
Another property of triangles is that the measure of an exterior angle of a
triangle is equal to the sum of the measures of the remote interior angles.
An exterior angle of a triangle is the angle formed by extending one of the
sides of the triangle past a vertex (the point at which two sides meet). An
exterior angle is always supplementary to the interior angle with which it
shares a vertex, and equal in measure to the sum of the measures of the remote
interior angles. Take a look at the figure below, in which d, the
exterior angle, is supplementary to interior angle c:

It doesn’t matter which side of a triangle you extend to create an exterior
angle; the exterior angle will always be supplementary to the interior angle
with which it shares a vertex and therefore (because of the 180º rule) equal to
the sum of the remote interior angles.
3. Triangle Inequality
The third important property of triangles is the triangle inequality rule, which
states: the length of a side of a triangle is less than the sum of the lengths
of the other two sides and greater than the difference of the lengths of the
other two sides.
Observe the figure below:

From the triangle inequality, we know that c – b < a < c + b. The exact
length of side a depends on the measure of the angle created by sides
b and c. If this angle is large (close to 180º) then a will be
large (close to b + c). If this angle is small (close to 0º), then a
will be small (close to b – c).
For an example, take a look at this triangle:

Using the triangle inequality, we can tell that 9 – 4 < x < 9 + 4, or 5 <
x < 13. The exact value of x depends on the measure of the angle
opposite side x.
4. Proportionality of Triangles
This brings us to the last basic property of triangles, which has to do with the
relationships between the angles of a triangle and the lengths of the triangle’s
sides. In every triangle, the longest side is opposite the largest angle and the
shortest side is opposite the smallest angle.

In this figure, side a is clearly the longest side and <A is the largest angle. Conversely, side c is the shortest side and
<C is the smallest angle. It follows, therefore, that c < b < a and C < B
< A. This proportionality of side lengths and angle measures holds true for
all triangles.
We did not assign measures and lengths to the angles and sides for the figure
above. If we had limited information about those values, however we could make
certain assumptions about the other unknown side lengths and angles measures.
For example, if we knew the measures of two of the angles in the triangle, we
could find the measure of the third angle and therefore decide which side is the
longest (it would be the side opposite the largest angle). This is the kind of
reasoning that you might have to use when dealing with triangles on the test.

|
|
|
|
|
What is one possible value of x if angle C < A < B? |
|
|
(A) |
1
|
|
|
(B) |
6
|
|
|
(C) |
7
|
|
|
(D) |
10
|
|
|
(D) |
15 |
Video Lesson - Types of Triagles
The largest angle in triangle ABC is <B ,
which is opposite the side of length 8. The smallest angle in triangle ABC
is <C, which is opposite the side of length 6. This means that the third side, of
length x, measures between 6 and 8 units in length. The value 7 is the
only choice that fits the criteria.
Special Triangles
There are several special triangles that have particular properties. Knowing
these triangles and what makes each of them special will help you immeasurably
on the test.
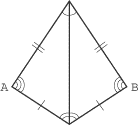
But before getting into the different types of special triangles, we must take a
moment to explain the markings we use to describe the properties of each
particular triangle. For example, the figure below has two pairs of sides of
equal length and three congruent angle pairs: these indicate that the sides have
equal length. The arcs drawn into <A and <B indicate that these angles are congruent.
In some diagrams, there might be more than one pair of equal sides or congruent angles. In this case, double hash
marks or double arcs can be drawn into a pair of sides or angles to indicate
that they are equal to each other, but not necessarily equal to the other pair
of sides or angles:
Now, on to the special triangles.
Scalene Triangles
A scalene triangle has no equal sides and no equal angles.

In fact, the special property of scalene triangles is that they don’t really
have any special qualities. Scalene triangles almost never appear on the Math
IC.
Isosceles Triangles
A triangle that contains two sides of equal length is called an isosceles
triangle. In an isosceles triangle, the two angles opposite the sides of
equal length are congruent. These angles are usually referred to as base angles.
In the isosceles triangle below, side a = b and <A = B

If you know the value of one of the base angles in an isosceles triangle, you
can figure out all the angles. For example, if one base angle of an isosceles
triangle is 35º, then you know that the other base angle is also 35º. Since the
three angles in a triangle must add up to 180º, you can figure out the value of
the third angle: 180º – 35º – 35º = 110º.
Equilateral Triangles
A triangle whose sides are all of equal length is called an equilateral
triangle. All three angles in an equilateral triangle are congruent as well;
the measure of each is 60º.

If you know that a triangle has three equal sides, then the proportionality rule
states that the triangle must also have three equal angles. Similarly, if you
know that a triangle has three equal angles, then you know it has three equal
sides.
Right Triangles
A triangle that contains a right angle is called a right triangle. The
side opposite the right angle is called the hypotenuse of the right triangle,
and the other two sides are called legs. The angles opposite the legs of a right
triangle are complementary.
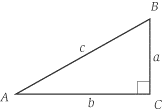
s
In the figure above,<C is the right angle (as indicated by the box drawn in the angle), side c
is the hypotenuse, and sides a and b are the legs.
The Pythagorean Theorem
The Pythagorean theorem is crucial to answering most of the right-triangle
questions that you’ll encounter on the test. The theorem will also come in
handy later on, as you study coordinate geometry and trigonometry. The theorem
states that in a right triangle a2 + b2 =
c2:

where c is the length of the hypotenuse, and a and b are
the lengths of the two legs. The theorem states that the square of the
hypotenuse is equal to the sum of the squares of the two legs.
If you know the measures of two sides of a right triangle, you can always use
the Pythagorean theorem to find the third.
Pythagorean Triples
Because right triangles obey the Pythagorean theorem, only a few have side
lengths which are all integers. For example, a right triangle with legs of
length 3 and 5 has a hypotenuse of length v32 + 52 = v9 + 25 =
v 34 = 5.83 .
The few sets of three integers that do obey the Pythagorean theorem and can
therefore be the lengths of the sides of a right triangle are called Pythagorean
triples. Here are some common ones:
{3, 4, 5}
{5, 12, 13}
{7, 24, 25}
{8, 15, 17}
In addition to these Pythagorean triples, you should also watch out for their
multiples. For example, {6, 8, 10} is a Pythagorean triple, since it is a
multiple of {3, 4, 5}.
The Math IC is full of right triangles whose side lengths are Pythagorean
triples. Study the ones above and their multiples. If you can recognize a
Pythagorean triple on a triangle during the test, you can drastically reduce the
amount of time you need to spend on the problem since you won’t need to do any
calculations.
Special Right Triangles
Right triangles are pretty special in their own right. But there are two
extraspecial right triangles that appear frequently on the Math IC. They are
30-60-90 triangles and 45-45-90 triangles.
30-60-90 Triangles
A 30-60-90 triangle is a triangle with angles of 30º, 60º, and 90º. What makes
it special is the specific pattern that the lengths of the sides of a 30-60-90
triangle follow. Suppose the short leg, opposite the 30 degree angle, has length
x. Then the hypotenuse has length 2x, and the long leg, opposite
the 60 degree angle, has length x v3.
The sides of every 30-60-90 triangle will follow this ratio of 1 : 2 : v3.

The constant ratio of the lengths of the sides of a 30-60-90 triangle means that
if you know the length of one side in the triangle, you will immediately know
the lengths of all the sides. If, for example, you know that the side opposite
the 30º angle is 2 meters long, then by using the 1 : 2 : v3
ratio, you will know that the hypotenuse is 4 meters long, and the leg opposite
the 60º angle is 2 v3
meters. On the Math IC, you will quite often encounter a question that will
present you with an unnamed 30-60-90 triangle, allowing you to use your
knowledge of this special triangle. You could solve these questions by using the
Pythagorean theorem, but that method takes a lot longer than simply knowing the
proper 30-60-90 ratio.
45-45-90 Triangles
A 45-45-90 triangle is a triangle with two 45º angles and one right angle. This
type of triangle is also sometimes referred to as an isosceles right triangle,
since it’s both isosceles and right. Like the 30-60-90 triangle, the lengths of
the sides of a 45-45-90 triangle also follow a specific pattern that you should
know. If the legs are of length x (they are always equal), then the
hypotenuse has length x v2.
Take a look at this diagram:

As with 30-60-90 triangles, knowing the 1: 1: v2
ratio for 45-45-90 triangles can save you a great deal of time on the Math IC.
Similar Triangles
Two triangles are called similar if the ratio of the lengths of their
corresponding sides is constant. In order for this to be true, the corresponding
angles of each triangle must be congruent. In essence, similar triangles have
exactly the same shape but not necessarily the same size. Take a look at a few
similar triangles:

Video Lesson - Similar Triangles
As you may have assumed from the above figure, the symbol for “is similar to” is
~. So if triangle ABC is similar to triangle DEF, you will write
ABC ~ DEF.
When you say that two triangles are similar, it is important to know which sides
of each triangle correspond to each other. After all, the definition of similar
triangles is that “the ratio of the lengths of their corresponding sides is
constant.” So, considering that ABC ~ DEF, you know that the ratio
of the short sides equal the ratio of the larger sides.
AB/DE = BC/EF =
CA/FD.
Just as similar triangles have corresponding sides, they also have corresponding
angles. If ABC ~ DEF, then <A = n <D ,n <B =n <E , and n <C=n <F.
Occasionally, the Math IC may present you with two separate triangles and tell
you that the two are similar. More often, the Math IC will present you with a
single triangle that contains a line segment parallel to one base. This line
segment creates a second, smaller, similar triangle. In the figure below, for
example, line segment DE is parallel to CB, and triangle ABC
is similar to triangle AED.

After presenting you with a diagram like the one above, the Math will test
whether you understand similarity by asking a question like:
If BC = 6 and AD = 2/3 AC what is DE ?
This question doesn’t tell you outright that DE and CB are
parallel, but it implicitly tells you that the two lines are parallel by
indicating that both lines form the same angle, xº, when they intersect
with BA. Once you realize that ABC ~ AED, you know that the
corresponding sides of the two triangles are in constant proportion. The
question tells you what this proportion is when it tells you that AD =
2/3 AC. To solve for DE, you have to plug it
into the proportion along with CB:

Area of a Triangle
It’s quite likely that you will have to calculate the area of a triangle for the
Math IC. The formula for the area of a triangle is:
A = 1/2 bh
where b is the length of a base of the triangle, and h is height
(also called the altitude).
Video Lesson - Area of Trangle
In the previous sentence we said “a base” instead of “the base” because you can
actually use any of the three sides of the triangle as the base; a triangle has
no particular side that is the base until you designate one. The height of the
triangle depends on the base, which is why the area formula always works, no
matter which side you choose to be the base. The heights of a few triangles are
pictured with their altitudes drawn in as dotted lines.

Study the triangle on the right. Its altitude does not lie in the interior of
the triangle. This is why the altitude of a triangle is defined as a line
segment perpendicular to the line containing the base and not simply as
perpendicular to the base. Sometimes the endpoint of the altitude does not lie
on the base; it can be outside of the triangle, as is the case of the second
example above.
On the Math , you may be tested on the area of a triangle in a few different
ways. You might be given the altitude of a triangle along with the length of the
base, but it’s unlikely you’d get such an easy question. It’s more probable that
the altitude would have to be found, using other tools and techniques from plane
geometry. For example, try to find the area of the triangle below:

To find the area of this triangle, draw in the altitude from the base (of length
9) to the opposite vertex. Notice that now you have two triangles, and one of
them (the smaller one on the right) is a 30-60-90 triangle.

The hypotenuse of this 30-60-90 triangle is 4, so the short side is 2 and the
medium side, which is also the altitude of the original triangle, is 2v3.
Now you can use the area formula to find the area of the original triangle:
1/2bh = 1/2(9)(2v3)
= 9v3
˜ 15.6.
Next to display next topic in the chapter.
Mathematics Practice Questions
Video Lessons and 10 Fully Explained Grand Tests
Large number of solved practice MCQ with explanations. Video Lessons and 10 Fully explained Grand/Full Tests.