Lesson: Chapter - 8

Explanations

1. A

If the pulley system doesn’t move, then the net force on both masses is zero. For mass m, that means that the force of gravity, mg, pulling it downward, is equal to the force of tension in the rope, pulling it upward. If the force of tension pulling mass m upward is mg, then the force of tension pulling mass M toward the edge of the table is also mg. That means that the force of static friction resisting the pull of the rope must also equal mg. The force of static friction for mass M is µ Mg, where µ is the coefficient of static friction. Since this force must be equal to mg, we can readily solve for µ:

2. C

The normal force is always normal, i.e., perpendicular, to the surface that exerts it, and in a direction such that one of its components opposes gravity. In this case, the inclined plane’s surface exerts the force, so the normal force vector must be perpendicular to the slope of the incline, and in the upward direction.

3. C

The acceleration of any particle due to the force of gravity alone doesn’t depend on the mass, so the answer is C. Whether or not the mass is on an inclined plane doesn’t matter in the least bit. We can prove this by calculating the acceleration mathematically:

F = ma = mg isn?
a = g sin?

As you can see, the acceleration depends only on the angle of the incline, and not on the mass of the block.

4. C

The system will be in equilibrium when the net force acting on the 1 kg mass is equal to zero. A free-body diagram of the forces acting on the 1 kg mass shows that it is in equilibrium when the force of tension in the pulley rope is equal to mg sin ?, where m = 1 kg and ? is the angle of the inclined plane.

Since the system is in equilibrium, the force of tension in the rope must be equal and opposite to the force of gravity acting on the 0.5 kg mass. The force of gravity on the 0.5 kg mass, and hence the force of tension in the rope, has a magnitude of 0.5 g. Knowing that the force of tension is equal to mg sin ?, we can now solve for ?:

mg sin? = 0.5 g
(1 kg) sin? = 0.5
? = 30°

5. ;D

The best way to approach this problem is to draw a free-body diagram:

From the diagram, we can see that there is a force of mg sin ? pulling the object down the incline. The force of static friction is given by µ N, where µ is the coefficient of static friction and NN is the normal force. If the object is going to move, then mg sin ? > µ N. From the diagram, we can also see that N = mg cos ?, and with this information we can solve for µ:

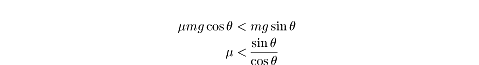
This inequality tells us that the maximum value of µ is sin? / cos?..

6. B

The velocity of a spring undergoing simple harmonic motion is a maximum at the equilibrium position, where the net force acting on the spring is zero.

7. D

The equilibrium position is the position where the net force acting on the object is zero. That would be the point where the downward force of gravity, mg, is perfectly balanced out by the upward spring force, kx, where k is the spring constant and x is the object’s displacement. To solve this problem, we need to equate the two formulas for force and solve for x:

8. ;D

The force of gravity always operates directly downward on the surface of the Earth. It doesn’t matter what other forces act upon the body. Thus the answer is D.

9. C

The forces acting upon the object in this diagram are tension and gravity. The force of tension is along the direction of the rod, in the direction of A. The force of gravity is directly downward, in the direction of D. The net force acting on the pendulum bob is the vector sum of these two forces, namely C.

10. E

Since the instantaneous velocity of the pendulum bob is in the direction of E, that is the path that the object will travel along. Eventually, the force of gravity will cause the pendulum bob to fall downward, but the question only asks you for the instantaneous velocity of the bob the moment it is released.

Next to display next topic in the chapter.