Lesson: Chapter - 8
Explanations
1.B
If you understand how to answer this question, you should be able to answer any
question dealing with line segments on the Math IC. The question asks you to
find the distance between two midpoints, so you first need to find where those
midpoints are. By definition, a midpoint is a point equidistant from both ends
of a line segment. So the midpoint of AD is 15 ÷ 2
= 7.5 units from either end. To find the midpoint of BC, you first need
to find the length of BC. From the figure, you can see that BC =
AD – AB – CD. Since it is given that AB + CD
= 2/5 AD,

The midpoint of BC is 9/2 = 4.5 units from B
and C. But in order to measure the distance between the midpoints of
BC and AD, you must situate the midpoint of BC with regard to
AD. You can do this by finding out the midpoint of BC’s distance
from point A.
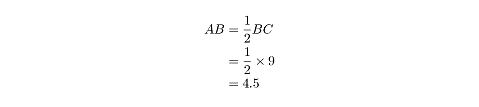
Therefore, the midpoint of BC is 4.5 + 4.5 = 9 units from a,
whereas the midpoint of AD is 7.5 units from a. The distance
between these two midpoints is 9 – 7.5 = 1.5.
div>
2.E
The slope of y = 3x + 4 is 3. A line perpendicular to this line
has a slope that is the opposite of the reciprocal of 3, or –1/3.
If the x-intercept of the desired line is 6, then the line contains the
point (6, 0), and we have enough information to put it in point-slope form: (y
– 0) = –1/3(x – 6). This simplifies to y = –1/3
x + 2.
3.A
The slope of the line pictured is –12/6 = –2. The y-intercept
of the line pictured is –2. So the equation of the line pictured is y =
–2x – 2. This leaves three choices. The line in the picture is dotted;
this means that the inequality is either < or >, not = or =. Now we have two
choices left. Because the region to the right and above is shaded, > is the
correct inequality, but just to make sure, plug in a point or two. In this case,
try the origin, (0,0). It is in the shaded region, and must therefore satisfy
the inequality. Which inequality does is satisfy? It satisfies y > –2x
– 2, so this is the correct choice.
4.D
In this equation, y is raised to the first power, and x is
squared. This should lead you to believe that the graph of this equation is a
parabola (in the equation of a circle, both variables are squared). Simplify the
equation to arrive at the standard form:

The equation in the question is the standard form of a parabola. From it we can
see that –3 < 0, so the parabola opens downward, and the vertex is (2, 6).
Back
Next
div>
Next to display next topic in the chapter.
Mathematics Practice Questions
Video Lessons and 10 Fully Explained Grand Tests
Large number of solved practice MCQ with explanations. Video Lessons and 10 Fully explained Grand/Full Tests.