Lesson: Chapter - 8

Lines

Lines are nothing more than an infinite set of points arrayed in a straight formation, but there are a number of ways to analyze them. We look at some of the main properties, formulas, and rules of lines.

Slope

The slope of a line is a measurement of how steeply the line climbs or falls as it moves from left to right. More technically, it is a line’s vertical change divided by its horizontal change, informally known as “the rise over run.” Given two points on a line, call them (x₁, y₁) and (x₂, y₂), the slope of that line can be calculated using the following formula:

So, for example, the slope of a line that contains the points (–2, –4) and (6, 1) is m = (1 – (–4)) / (6 – (–2)) = ⁵/₈.

Positive and Negative Slopes

You can easily determine whether the slope of a line is positive or negative just by looking at the line. If a line slopes uphill as you trace it from left to right, the slope is positive. If a line slopes downhill as you trace it from left to right, the slope is negative. You can get a sense of the magnitude of the slope of a line by looking at the line’s steepness. The steeper the line, the greater the slope will be; the flatter the line, the smaller the slope will be. Note that an extremely positive slope is larger then a moderately positive slope while an extremely negative slope is smaller then a moderately negative slope.

Look at the lines in the figure below and try to determine whether the slope of each line is negative or positive and which has the greatest slope:

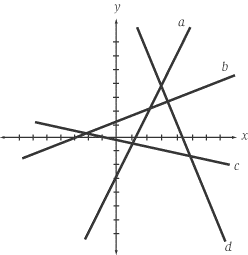
Lines a and b have positive slopes, and lines c and d have negative slopes. In terms of slope magnitude, line a > b > c > d.

Special Slopes

For the Math IC, there are a few slopes you should recognize by sight. If you can recognize one of these lines and identify its slope without having to do any calculations, you will save yourself a lot of time.

• A line that is horizontal has a slope of zero. Since there is no “rise,” y₂ – y₁ = 0, and thus m = ^y2–y1 /_x2–x1 = ⁰/_x2–x1 = 0.
• A line that is vertical has an undefined slope. In this case, there is no “run,” and x₂ – x₁ = 0. Thus, m = ^y2–y1 /_x2–x1 = ^y2–y1/ ₀, and any fraction with 0 in its denominator is, by definition, undefined.
• A line that makes a 45º angle with a horizontal line has a slope of 1 or –1. This makes sense because the rise equals the run, and y₂ – y₁ = x₂ – x₁, or y₂ – y₁ = –(x₂ – x₁).

Of the four lines pictured below, one has a slope of 0, one has a slope of 1, another has a slope of –1, and another has undefined slope. Decide which is which.

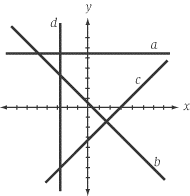
Line a has slope 0 because it is horizontal. Line b has slope –1 because it slopes downward at 45º as you move from left to right. Line c has slope 1 because it slopes upward at 45º as you move from left to right. Line d has undefined slope because it is vertical.

Parallel and Perpendicular Lines

Parallel lines are lines that don’t intersect. In coordinate geometry, they can also be described as lines with the same slope.

Perpendicular lines are lines that intersect at a right angle. In coordinate geometry, perpendicular lines have opposite, reciprocal slopes. That is, a line with slope m is perpendicular to a line with a slope of –¹/_m.

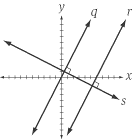
In the figure, lines q and r both have a slope of 2, so they are parallel. Line s is perpendicular to both lines q and r, and thus has a slope of –¹/₂.

Equation of a Line

A line in coordinate geometry can be described by an equation containing the variables x and y. For the Math IC, you need to understand thoroughly two forms of the equation of a line: the slope-intercept form and the point-slope form.

Slope-Intercept Form

The slope-intercept form of the equation of a line is:

where m is the slope of the line, and b is the y-intercept of the line. Both are constants.

The y-intercept of a line is the y-coordinate of the point where the line intersects the y-axis. Likewise, the x-intercept of a line is the x-coordinate of the point where the line intersects the x-axis. Therefore, if given the slope-intercept form of the equation of a line, you can find both intercepts.

For example, in order to find the y-intercept, simply set x = 0 and solve for the value of y. For the x-intercept, set y = 0 and solve for x.

To sketch a line given in slope-intercept form, first plot the y-intercept, and then use the slope of the line to plot another point. Connect the two points to form your line. In the figure below, the line y = –2x + 3 is graphed.

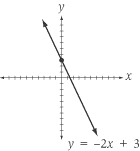
Since the slope is equal to –2, the line descends 2 units for every 1 unit it moves in the positive x direction. The y-intercept is at 3, so the line crosses the y-axis at (0, 3). For practice, solve for the x-intercept.

Point-Slope Form

The point-slope form of the equation of a line is:

where m is the slope of the line, and (x₁, y₁) is a point on the line.

The point-slope form and slope-intercept form are alternative ways of expressing the same equation. In fact, the slope-intercept form is the point-slope form taken at the y-intercept, or the point (0, y₁):

Since y₁ = b (the y-intercept is simply the y-coordinate of the point at which x = 0), the two forms are equal.

The slope-intercept form of the line equation is the more common of the two, but the point-slope form is useful when your only information is the slope and a point (hence the name point-slope form).

Example Problems with Slope

The Math IC test often asks questions that require you to understand the slope-intercept form and the point-slope form, and to be able to convert between the two.

What is the slope-intercept equation of the line that contains the point (3, 4) and is perpendicular to the line y = ¹/₃x – 6?

To answer this question, you first need to find the slope of the line whose equation you are trying to determine. Fortunately, the question gives you the slope of a perpendicular line, and we know that the slope of a line is the opposite reciprocal of the slope of the line to which it is perpendicular. Thus, the slope is –1/ (¹/₃) = –3. If the line contains the point (3, 4), its point-slope equation is y – 4 = –3(x – 3). To convert this to slope-intercept form, use algebra:

What is the slope-intercept form of the equation of the line that contains the points (5, 3) and (–1, 8)?

Start by finding the slope of the line. You can calculate the slope with the two points you’re given: m = ^8–3/_–1–5 = –⁵/₆. To put the equation of this line in slope-intercept form, the only additional information we need is the y-intercept. To find it, use the x- and y-coordinates of a point that you know is on the line and plug them into the equation y = –⁵/₆ x + b, and solve for b. Using the point (5, 3):

The slope-intercept form of the equation of this line is y = –⁵/₆ x + ⁴³/₆.

Next to display next topic in the chapter.

Mathematics Practice Questions