Tangent Lines
A line that intersects the circle at only one point is called a tangent
line. The radius whose endpoint is the intersection point of the tangent line
and the circle is always perpendicular to the tangent line.

Every point in space outside the circle can extend exactly two tangent lines to
the circle. The distance from the origin of the two tangents to the points of
tangency are always equal. In the figure below, XY = XZ.
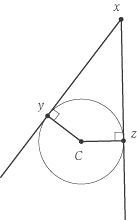
The Math IC often includes tangent lines in the test. For example:
What is the area of triangle
QRS, if
RS is tangent to circle
Q?
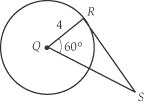
If RS is tangent to circle Q, then QR is perpendicular to
RS, and therefore QRS is a 30-60-90 triangle. Given that QR
= 4, we know that RS = 4 v3,
and the area of triangle QRS is 1/2(4)(4v3)
= 8v3.
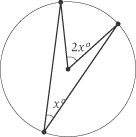
Central Angles and Inscribed Angles
An angle whose vertex is the center of the circle is called a central
angle.
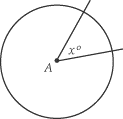
The degree of the circle (the slice of pie) cut by a central angle is equal to
the measure of the angle. If a central angle is 25º, then it cuts a 25º arc in
the circle.
An inscribed angle is an angle formed by two chords in a circle
that originate from a single point.
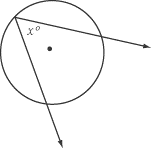
An inscribed angle will always cut out an arc in the circle that is twice the
size of the degree of the inscribed angle. If an inscribed angle has a degree of
40º, it will cut an arc of 80º in the circle.
If an inscribed angle and a central angle cut out the same arc in a circle, the
central angle will be twice as large as the inscribed angle.
Circumference of a Circle
The circumference of a circle is the length of the 360º arc that forms
the circle. In other words, if you were to trace around the edge of the circle,
it is the distance from a point on the circle back to itself. The circumference
is the perimeter of the circle. The formula for circumference is:
C = 2pr
where r is the radius. The formula can also be written C = pd,
where d is the diameter. Using the formula, try to find the circumference
of the circle below:
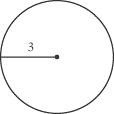
Plugging the radius into the formula, C = 2p
r = 2p (3) = 6p.
Arc Length
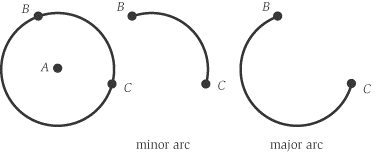
An arc is part of a circle’s circumference. An arc contains two endpoints
and all the points on the circle between the endpoints. By picking any two
points on a circle, two arcs are created: a major arc, which is by definition
the longer arc, and a minor arc, which is the shorter one.
Since the degree of an arc is defined by the central or inscribed angle that
intercepts the arc’s endpoints, you need only know the measure of either of
those angles and the measure of the radius of the circle to calculate the arc
length. The arc length formula is:
arc length = n/360 × 2pr
where n is the measure of the degree of the arc, and r is the
radius. The formula could be rewritten as arc length = n/360× C, where C is the circumference of the circle.
A Math IC question might ask:
Circle
D has radius 9. What is the length of arc
AB?
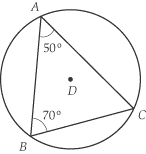
In order to figure out the length of arc AB, you need to know the radius
of the circle and the measure of <C,
which is the inscribed angle that intercepts the endpoints of AB. The
question tells you the radius of the circle, but it throws you a little
curveball by not providing you with the measure of <C.
Instead, the question puts <C in a triangle and tells you the measures of the other two angles in the
triangle. Using this information you can figure out the measure of <C.
Since the three angles of a triangle must add up to 180º, you know that:
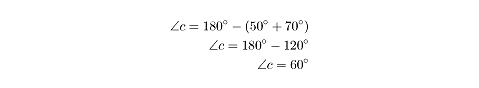
Since angle c is an inscribed angle, arc AB must be 120º. Now you
can plug these values into the formula for arc length
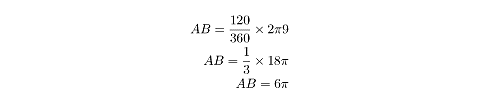
Area of a Circle
The area of a circle depends on the radius of the circle. The formula for area
is:
Area = pr
2
where r is the radius. If you know the radius, you can always find the
area.
Area of a Sector
A sector of a circle is the area enclosed by a central angle and the
circle itself. It’s shaped like a slice of pizza. The shaded region in the
figure below is a sector:
The area of a sector is related to the area of a circle the same way that the
length of an arc is related to circumference. To find the area of a sector,
simply find what fraction of 360º the sector comprises and multiply this
fraction by the area of the circle.
Area of Sector =
n/360 × p
2
where n is the measure of the central angle which forms the boundary of
the sector, and r is the radius.
Try to find the area of the sector in the figure below:
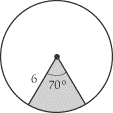
The sector is bounded by a 70º central angle in a circle whose radius is 6.
Using the formula, the area of the sector is:
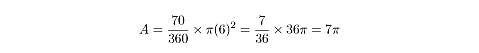
Polygons and Circles
You could potentially see a question or two on the Math IC that involve polygons
and circles in the same figure. Here’s an example:
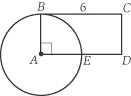
What is the length of major arc BE if the area of rectangle ABCD
is 18?
To find the length of major arc BE, you must know two things: the measure
of the central angle that intersects the circle at its endpoints and the radius
of the circle. Because ABCD is a rectangle, and rectangles only have
right angles, figuring out the measure of the central angle is simple.
<BAD is 90º, so the measure of the central angle is 360º – 90º = 270º.
Finding the radius of the circle is a little tougher. From the diagram, you can
see that it is equal to the height of the rectangle. To find the height of the
rectangle, you can use the fact that the area of the rectangle is 18, and the
length is 6. Since A = bh, and you know the values of both a and
b,
h= A ÷ b
= 18 ÷ 6
= 3
With a radius of 3, we can use the arc length formula to find the length of
major arc BE.
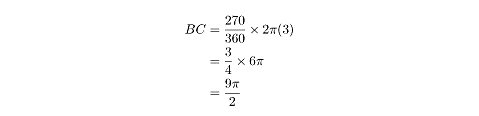 Back
Next
Back
Next
Next to display next topic in the chapter.
Mathematics Practice Questions
Video Lessons and 10 Fully Explained Grand Tests
Large number of solved practice MCQ with explanations. Video Lessons and 10 Fully explained Grand/Full Tests.